Resolving Bankruptcy Claims
Posted March 2005.
There are surprisingly many approaches to finding ways of distributing money to bankruptcy claimants...
Joe Malkevitch
York College (CUNY)
malkevitch at york.cuny.edu 
Introduction
New companies are born regularly. They represent the optimism of their creators that they can market a product or service better than others. Such companies raise capital and open their doors, or at least as likely these days, their cell phones and computers. They might hire workers, rent space, and order things from other businesses. If all goes well these companies thrive and are successful, but all too often things turn sour and the companies suffer a cash flow problem. They have too many creditors and not enough money in the bank. So the company goes bust - bankrupt. This means that while the company may have some assets, the value of these assets is exceeded by the claims against these assets. This situation is handled by laws in every individual country. However, this situation also gives rise to very interesting mathematics. It turns out there are surprisingly many approaches to finding ways of "distributing money" to the claimants which obey a variety of equity and fairness principles. Here is a two-person example where, unless the problem is carefully chosen, it is common for the different methods to yield the same solutions. However, with more claimants, different methods are more likely to yield different solutions.
Suppose E (remaining assets with which to settle claims) = $210
Claimant 1 has verified claims of $300
Claimant 2 has verified claims of $60.
How many different seemingly fair ways can you find to solve this problem? More specifically, your job is to find two numbers, the amounts to be given to each of the claimants, whose sum is $210.
Basic ideas
In the simplest form of a bankruptcy problem we have a collection of claimants: C1, C2, C3, ...., Cn, with verified claims c1, c2, c3, ..., cn. The remaining assets E have also been verified and are to be distributed by a "wise person" or judge. (The letter E is used to suggest the word "estate.") If someone leaves a will, it may turn out that the estate is not large enough to make the suggested dispersals. In this case, we have a "bankruptcy" problem where we treat the desired amounts to be dispersed as the claimant amounts and E is to be used to pay off these claims. (Later, I will mention a variety of ways that other problems can be recast in ways that either resemble bankruptcy problems or where the ideas used in studying bankruptcy problems provide insight into these other questions. In the recent economics literature, bankruptcy problems are often defined in a narrower way than I am using here.) Assume that the amount to be distributed is strictly less than the amount which is being claimed. Thus, c1 + c2 + c3 + .... + cn > E. We would like to be able to advise the judge about how to distribute the money using the best insights about fairness and equity principles. We are assuming that the claimants are isolated from each other and do not "bargain" or "negotiate" with each other regarding the amounts they might get from the judge. One can imagine that one could have a "game" where the claimants would get nothing if they could not agree how to split the estate, but if they could agree to share all of E in some way, they would be collectively allocated this amount. In this type of situation negotiations among the claimants would be required.
Total equality
Perhaps the first approach to solving equity problems is to treat individuals with "total equality." It is this thought that governs the famous dictum with regard to voting: "one person, one vote." If we do this in our example, we take the estate E, which amounts to $210 and divide by 2, giving $105. This amount would be given to each of the claimants. This may seem strange because we are not taking into account the size of the claims to get this value, only the number of claimants and the value of E being used. In particular, for the numbers here this means giving Claimant 2 more than he/she claimed! Can you think of a real world situation where this might actually be reasonable?
Should we decide that the above method is unreasonable, we might adopt the following second method: Equalize the claims of the claimants as much as possible but never give a claimant more than is requested. This notation of solving a bankruptcy has very old roots, having been in essence suggested by the great medieval philosopher Moses Maimonides (1135-1204) (often referred to only as Maimonides or as Rambam).
The method of Maimonides
In modern mathematical terminology what we have here is a constrained optimization problem. Our desire is to make the amount given to each of the claimants as equal as possible but not to have any claimant receive more than his/her claim. This means in the mathematical formulation that certain inequalities would have to hold for a solution. Here is a geometrical way to solve this kind of problem easily without converting it to highly symbolic mathematical form. Imagine that the money in the estate to be distributed is a blue fluid. We begin to fill up two "bins" or "tanks" of size 300 and 60 (the claim sizes) with a small bit of fluid in each, keeping the amounts as equal as possible, as illustrated in Figure 1 below. Remember that the size of the tanks corresponds to the size of the claims.
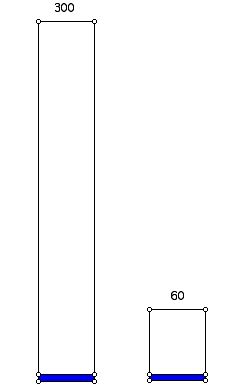
Figure 1
We keep filling the two containers equally until we fill up the smaller of the two bins, which amounts to completely filling this claim. The situation is now as shown in Figure 2 below:

Figure 2
How much of the estate has been used up at this stage? The answer is 2(60) or $120. This leaves $210 - $120 = $90 to distribute and this all goes to Claimant 1 since the complete claim of Claimant 2 has been met. Since $60 + $90 is $150 the final settlement gives Claimant 1 $150 and Claimant 2 $60. Figure 3 shows this solution:
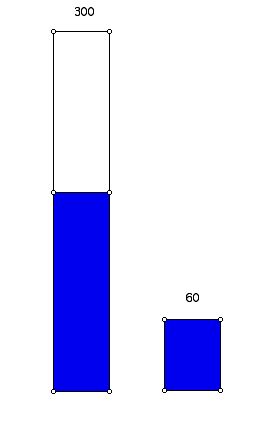
Figure 3
This geometric approach works very well for a large number of claimants. Typically the claim of the smallest claimant can be fulfilled and then if there is more "estate fluid" to distribute this is done until the next smallest claimant's claim is fulfilled. The process continues until all the estate "fluid" is gone.
Loss methods
Are you happy with the Maimonides solution to the bankruptcy problem? Unlike the total equality solution, it takes into account the size of the claims . However, let us look, for example, at how much of what each claimant hoped for, failed to get recovered. For C2 this amount is 0, while for C1 this amount is $150. This does not seem to spread the pain of "loss" very fairly. How much do the claimants collectively lose? Since E = $210 and the claimants are claiming $300 and $60 respectively, the loss L = $360 - $ 210 = $150. This notion suggests a new method. Why not spread the loss equally? This would mean assigning a loss of $75 to each claimant. For C1 this amounts to giving him/her $300 - $75 or $225, while for C2 this amounts to giving him/her $60 - $75 = - $15! Although $225 + (-$15) adds to $210, the amount E the judge must distribute, something seems wrong here! The problem is that Claimant 2 is being asked to "subsidize" the settlement. The -$15 that Claimant 2 coughs up is given to Claimant 1 along with all of the $210 available to the judge. This total of $225 makes it possible to cut Claimant 2's loss to $75, which is equal to that of Claimant 1. However, many people will consider this unfair because the pain of Claimant 2 is made worse by having to subsidize the settlement. (Can you think of a real world situation where it might not seem totally unreasonable to ask players to subsidize the settlement of the bankruptcy to achieve some goal?)
Like the contrast between "total equality" and Maimonides, one can consider the analogue for loss of Maimonides. The idea is to equalize loss as much as possible without any claimant's loss becoming negative as a result. To do this we must reduce the loss of the player with the largest claim to that of the person with the second largest claim, if this is possible. In this case, if we give C1 $210 this will bring his loss to only $90; to reduce the loss further requires more money than is available in E. Thus, we accept the solution of c1 = $210 and c2 = $0.
Suppose we have three claimants with claims of $100, $80, and $60, and there is an estate E of $210. We can give $20 to the first claimant reducing his/her he "current loss" to $80. Now we can give $20 to each of claimants 1 and 2 which reduces all the claimants to a current loss of $60. At this point $60 of the estate has been used. This leaves $210 - $60 = $150. By giving each claimant $50 of this we can equalize the losses. Thus Claimant 1 gets $90, Claimant 2 gets $70 and Claimant 3 gets $50. These numbers add to $210 as required and give each claimant a loss of $10. Of course, in this problem one can also conceptualize as follows. Since the claims are $240, and E = $210, each claimant of the three will sustain a loss of $10. This means that $10 less than each claim is given to the claimant.
Proportional methods
Another natural approach to settling a bankruptcy is to award the claimants an amount proportional to their claims. This would entail in our prime example giving C1 the amount (300/360)(210) = $175 and C2 the amount (60/360)(210) = $35. This seems a very natural approach because it uses the size of the claims to decide how to divide what is given to each claimant. We might also look at settling the bankruptcy by proportionality of loss. The loss in this example is $150. Computing C1's loss we get (5/6)(150) = $125 and C2's loss would be (1/6)(150) = $25. Thus, we would have c1 = $300 - $ 125 = $175 and c2 = $60 - $25 = $35. This is the same solution as when we assign the gains proportionally. Is this an accident? No! We can use a bit of algebra to see that this result holds in general.
This is a nice example of how algebra can be used to prove a result which one might intuit is true by examples but requires the algebra to show that it is true in general. In fact, it turns out that one of the reasons that proportionality is an appealing approach to settling bankruptcy problems is that a variety of different points of view lead to the same solution. For example, another perspective on solving bankruptcy is to pay each claimant a fixed amount on each dollar for the claimant's loss. (Often after a fire destroys property, one hears that the insurance company paid off the losses that were incurred, for a certain amount per dollar.) For example, one might hear that a settlement involved paying 10 cents on the dollar. How can one determine the fixed equal percentage that claimants can get for our example? Suppose we let x represent that fixed percentage. Then we have that
Since (7/12)(300) = $175, and (7/12)(60) = $35 we see that the proportional solution gives the same solution as the "fixed percentage on the dollar" solution for this example. This situation is again not an accident; it holds in general.
I few years ago I developed what appears to be a new viewpoint which leads to the proportional solution. Since the amount E is not enough to pay off the bankruptcy, one might adopt the following point of view: Instead of giving the claimants less than they are entitled to now, one can postpone paying them off and wait until the available money E grows, by investing it at the current interest rate until the invested amount plus interest totals the amount being claimed. The judge at this future point in time would pay off each claimant his/her full amount. Using the well-known accounting principle of computing the present value of this future asset we can see what amount of money this approach would yield each claimant today. If one does the algebra involved, one sees that the solution is the same as the proportional solution. Further insights stem from thinking about the consequences of changing interest rates when one looks at bankruptcy from a time versus money tradeoff viewpoint. After all, interest rates do not stay constant for long.
More solution concepts
Having seen so many different approaches to settling bankruptcies, it might seem that we had exhausted the intuitively appealing ways to solve such problems. In fact, this turns out not to be true. Here are a few more interesting approaches to these questions.
Shapley Value
Another important solution idea for bankruptcy problems is based on a truly central solution concept for n-person games that is due to Lloyd Shapley, and is known as the Shapley Value. There are various ways to think of the Shapley Value but here I will work out an example which involves three rather than two claimants to make the ideas for one approach clearer. Assume that the claims are $120, $60, and $30 respectively, and the size E of the estate is $150. Imagine that the judge announces that the claimants should line up to get a share of the funds. Suppose the claimants form a line in front of the judge in the order C1C2C3.
Now what will the judge do with the line in front of her? She gives out the money being claimed for as long as the money that she has lasts. Thus, for the order above she gives the first claimant $120, the claimant C2 $30, because this is all of the $60 claim that can still be met, and the third claimant gets nothing because the funds are exhausted. Now it hardly seems fair to reward the particular line which formed in front of the judge, perhaps reflecting the speed with which the claimants mobilized themselves. However, we can consider all possible lines that might have formed, and use as the settlement the average of the amounts that claimant gets, where the average is taken over all of the line orders that might appear in front of the judge. Since there are 3 claimants, there are 6 different orders that the claimants might have formed in front of the judge. The table below shows the results of distributing the money for these 6 orders, and then averaging (taking the mean) over all 6 cases.
| |
C1
|
C2
|
C3
|
|
C1C2C3
|
120
|
30
|
0
|
|
C1C3C2
|
120
|
0
|
30
|
|
C2C1C3
|
90
|
60
|
0
|
|
C2C3C1
|
60
|
60
|
30
|
|
C3C1C2
|
120
|
0
|
30
|
|
C3C2C1
|
60
|
60
|
30
|
|
Mean
|
$95
|
$35
|
$20
|
While the Shapely Value can be found relatively easily for small games, the computational work for large games is considerable.
What solution does the Shapely Value give for the two-person example that we have been using all along? The table for this case is shown below:
| |
C1
|
C2
|
|
C1C2
|
210
|
0
|
|
C2C1
|
150
|
60
|
|
Mean
|
$180
|
$30
|
Contested garment rule
Finally, let us jump from the relatively recent Shapley Value to a solution concept that goes back hundreds of years. This solution idea is discussed in a "document" known as the Babylonian Talmud, which initially consisted of oral materials handed down from one generation to another. (There is also a Jerusalem Talmud.). It was Barry O'Neil who called attention in modern times to the fact that the Babylonian Talmud treats various examples of bankruptcy problems. In modern accounts the technique described in the Talmud has come to be known as the contested garment rule, since the method was applied to a situation where two individuals claimed portions of a single garment.
How does the contested garment rule work? The basic idea is that depending on the amounts of the claims and size of E, sometimes one or both of the claimants can argue that some of the money "belongs" to that claimant. For example, in our situation Claimant 1 goes to the judge and says, since the only other claimant is only asking for $60 and you have $210 available, $150 of your $210 should be awarded to me. Suppose we refer to this $150 as Claimant 1's "uncontested" claim against Claimant 2. Claimant 2 might try to argue in a similar vein, but in this case since Claimant 1 is claiming $300, Claimant 2 has no non-zero uncontested claim against Claimant 1. Now there remains $60 that both are claiming. Thus, the judge splits this amount equally between them. Hence, Claimant 1 gets $150 + $30 = $180 and Claimant 2 gets $0 + $30 = $30. On first hearing this seems like a strange approach but it also has a certain appeal! (Suppose Claimant 1 and Claimant 2 claim respectively $100 and $80 and E = $140. Claimant 1's uncontested claim against Claimant 2 is $60, while Claimant 2's uncontested claim against Claimant 1 is $40. Hence, the total of uncontested claims is $60 + $40 which means there is $40 remaining that both claim. The judge splits this evenly. Claimant 1 gets $60 + $20 = $80 and Claimant 2 gets $40 + $20 = $60.) It may not even be obvious that the sum of the uncontested claims is always less than the amount E but it always will be.
To help understand the contested garment rule better let us use a bit of analytical geometry (Figure 4). Suppose we plot along the horizontal axis the amount we might give to Claimant 1 and along the vertical axis the amount we might give to Claimant 2. We introduce coordinates with A having the coordinates (0, 0). There are some obvious rationality constraints that must be imposed. The amount given Claimant 1 must be less than or equal to $300 and the amount given to Claimant 2 must be less than or equal to $60. These requirements involve the segments FG and GC in our diagram below. These two conditions are sometimes referred to as "individual rationality" constraints. There is also an efficiency condition or group rationality condition, namely, that we must give away to the claimants collectively $210. This is represented by the line segment EB. The points where both individual rationality below FG and to the left of GC and group rationality hold are indicated by the red segment. Point J represents the midpoint of the red segment. This point corresponds to the contested garment solution in this example. One can prove that this will hold in general. The points in red are known to game theorists as the core points for when one treats this bankruptcy problem as a "game."

Figure 4
Notice that the contested garment rule in this two-claimant example gives the same solution as the Shapley Value. This is not an accident. The two solutions will always coincide for two claimants.
A mathematical detective story
One of the most interesting episodes in the modern understanding of bankruptcy problems arises from a detective story related to the ideas in the preceeding discussion concerning bankruptcy ideas originating in the Talmud. Robert Aumann and Michael Maschler, Israeli game theorists, were the mathematical detectives in this case. Biblical scholars presented them with the issue of explaining the data in the following table. The source of the data is the Talmud. Note there are three questions where the size of the claims are held fixed (columns) but the size of the estate varies from 100, to 200, and then to 300 (rows). The Talmud offers the numbers in the "body" of the table as the proposed answers.
|
|
Claim |
100
|
200
|
300
|
| Estate |
100
|
33 1/3
|
33 1/3
|
33 1/3
|
|
|
200
|
50
|
75
|
75
|
| |
300
|
50
|
100
|
150
|
The biblical scholars could make sense of the first and last lines of the table but were puzzled by the middle line. What was the method being used here? Was there a "copying error?" Over the years had some data been changed which resulted in the second line's being erroneous? The story is that initially Aumann and Maschler did not see how to explain the second line. However, to avoid a hasty conclusion they had a game theory package compute different solutions to various games. To their surprise one of the solution concepts coincided with the second line! This solution concept is known as the nucleolus.
The original definition of nucleolus was given in 1969 by David Schmeidler, who did his doctoral work at Hebrew University in Jerusalem and now is at Ohio State University. It grows in a natural way out of solution concepts of n-person games which for general games are rather complex. It seemed unlikely that early scholars were using these ideas as far back as when the Babylonian Talmud was put together. Aumann and Maschler realized that perhaps there was an approach to the nucleolus for the special class of games that arose in bankruptcy problems that would have made it possible to solve them with a clever use of arithmetic skills. Eventually they found a line of reasoning that did just that. The place they began was in the Middle Ages, when thoughts about bankruptcy centered not around the use of the proportional solution but along the lines of the contested garment rule. They knew that Maimonides and other scholars were concerned with both the gain and loss that a claimant received. Aumann and Maschler realized that viewed through a modern perspective, for every solution concept that involved gain there was a "dual" method which involved loss.
What Aumann and Maschler accomplished represented new mathematics in several ways. First, they discovered a new way to characterize the nucleolus as it applied to bankruptcy games.
Theorem: The nucleolus of a bankruptcy game divides the estate E among the claimants in such a way that the amount given to any two players can be computed as the contested garment solution of the two-player bankruptcy problem with the original claims of these players and using as an estate the amount given to these two individuals.
Next, Aumann and Maschler found an algorithm that finds the amounts to give each claimant which satisfy the property stated in the theorem. This method, which was previously unknown, has the property that for bankruptcy games, it computes the nucleolus in a way that could have been carried out by people who posed the bankruptcy problems found in the Babylonian Talmud.
Here is the algorithm found by Aumann and Maschler, which can be applied to the case of any number of claimants.
Step 1. Divide each of the claims by two, and use the Maimonides method to fill these (divided) claims. If the estate is exhausted, the algorithm terminates; otherwise go to step 2.
Step 2. Use the Maimonides loss method and the remaining amount from the estate to pay off the other half of the claims.
Here is an example:
We have three claimants C1, C2, and C3 whose claims are $180, $90, and $30 respectively. The value of the estate E is $240.
First, we divide the claims in half, and apply Maimonides' method. Since the claims are $90, $45 and $15, whose sum is $150, we can meet all of these claims with $240, leaving $90 left to try to equalize losses for the amounts of $90, $45, and $15. To do this we first bring down Claimant 1's loss to $45 by giving this claimant $45. We now must try to bring Claimant 1 and Claimant 2's loss down to the same level as Claimant 3. This can not be accomplished but we can reduce the losses further by giving each of Claimant 1 and Claimant 2 an additional $22.50. Hence, we give Claimant 1 $90 + $45 + $22.50 = $157.50, Claimant 2 $45 + $22.50 = $67.50 and Claimant 3 $15. This accounts for $240 as required. To check that this result is consistent with the contested garment rule for pairs of claimants we need to check three different problems:
a. Claimant 1 $180, Claimant 2 $90 and E = $225
b. Claimant 1 $180, Claimant 3 $30 and E = $172.50
c. Claimant 2 $90, Claimant 3 $30 and E = $82.50.
Let us check one of these cases, say, b.
Claimant 1's uncontested claim against Claimant 3 is $142.50 and Claimant 3's uncontested claim against Claimant 1 is $0. Thus, the judge has left $30 to split between the two, resulting in the settlement of Claimant 1 getting $157.50 and Claimant 2 getting $15.
You should check that, amazingly, the other two cases can be verified as well. Thus, with this very simple to understand approach, we can find a solution to a bankruptcy situation with any number of claimants, so that the amounts given to pairs of players split as required by the contested garment rule with the original claims of this pair!
To convert a bankruptcy problem to a game it is necessary to represent the game in one of a variety of ways. One standard way to represent a game is to specify for each coalition of players that might act together, what the payoff would be to that coalition. This form of representation for a game goes back to John Von Neumann, one of the pioneers with Oskar Morgenstern of the modern form of game theory. It was in this form that Aumann and Maschler realized the key role that the nucleolus played. Based on their seminal work of 20 years ago and that of O'Neil, other scholars have significantly extended our insights into bankruptcy games and related problems. For example, one can think of taxation collection as a bankruptcy problem. Thus, if the government needs to raise $E by taxation and there are "income classes" which have wealth to be taxed that have a sum greater than E, the amount of tax to be collected from each class plays the role of the amounts assigned to the claimants in a bankruptcy situation!!
Axiomatics
When people think of the power of axiom systems they invariably think of Euclid's Elements. The Elements set in motion a mathematical paradigm where, starting from a few basic undefined terms and rules (axioms), a large system of theorems was deduced using basic ideas of logic. It was one of the great milestones in intellectual history when Janos Bolyai and N. Lobachevsky independently showed that substituting a different axiom for Euclid's famous parallel postulate resulted in an alternative geometric world to Euclidean geometry.
Another such milestone was achieved by Kenneth Arrow, educated as an undergraduate mathematics major at City College (now a part of City University of New York). He went on to study mathematically related areas at Columbia University under the direction of Harold Hoteling and Abraham Wald. Arrow's axiomatic work involved voting and election decision systems. Before Arrow, more or less, scholars had sought appealing election and voting methods which they hoped to show had desirable properties.

Kenneth Arrow turned the situation on its head by writing down appealing fairness axioms and examining which election and voting methods obeyed his axioms. He showed that based on some reasonable assumptions there was no method that obeyed all the desirable conditions. Equally important, he inspired other workers to find fairness principles (axioms) which were uniquely obeyed by particular methods. This pattern of moving from intuitively appealing algorithms or techniques for solving fairness problems to finding axioms that characterize individual methods has become standard methodology. This approach has been followed for apportionment problems, fair division problems, bargaining problems (game theory solution concepts in general), and bankruptcy. New fairness principles to study are being developed all the time and new characterizations of important methods are being found.
Here are some examples of the kinds of fairness conditions that one might want a method that solves a bankruptcy problem to obey:
a. Symmetry
If two claimants have identical claims, then they should get identical amounts in the settlement of their claims.
Comment: This means that one is not taking into account, for example, the relative wealth of the claimants. A poor claimant with a large claim will get treated the same as a wealthy claimant with the same size claim.
b. Estate monotonicity
If a bankruptcy problem is solved for an estate size and a particular set of claims, and another bankruptcy problem is solved where the claims are the same size as in the first case, but the estate is now larger, then the amounts given to each claimant must be at least as large in the second case as in the first.
Comment: As the estate grows and the claims stay fixed, the amount that a claimant gets should never go down. It does seem reasonable that a claimant's settlement might not go up just because the estate increased in size from a small estate.
c. Newly found funds
Suppose we divide the estate E into two parts E' and E" where E = E' + E". Suppose we use our rule to settle the claims using E' as the estate, and then we use the same rule to settle these same claims using E". We would like the method when applied to E to give the same result as adding the results of applying the rule to E' and E" separately.
d. Claimant splitting
Suppose we have a pair of partners who collectively claim $1000. Suppose further that these partners submit separate claims of $600 and $400. We would like the bankruptcy solution not to give the split claimants more than the original claimant would have gotten. (A similar issue applies if groups of claimants who merge their claims do collectively better.)
Comment: If this axiom does not apply, it tempts claimants either to form one claimant from a group of claimants or to split into a group of claimants, in the hopes of getting more by this action.
Consider, for example, an estate of 40 to be split among individual claims of 100, 30, 10. Using Maimonides one sees that these claimants get 15, 15, and 10 respectively. However, if the person claiming 30 can argue that in fact there are separate claims of 20 and 10, and thus four claimants with 100, 20, 10, and 10, the "split claimant" gets more. Thus, Maimonides' method settles these claims at 10, 10, 10, and 10 and the "split claimant" gets 20 instead of 15.
William Thomson of the Department of Economics at Rochester University has collected and analyzed a great variety of different axioms that can be applied to bankruptcy problems.

Thomson's goal, like that of other game theorists, mathematicians, and economists who study bankruptcy problems, has been to get a list of axioms which characterize individual solution methods and families of methods for bankruptcy problems. Since no method can obey all the fairness axioms for bankruptcy problems that one might like to have satisfied, Thomson's efforts have gone far in clarifying the tradeoffs between different choices. At this point quite a few important methods have one or more characterizations. New models of bankruptcy and new axioms continue to be explored.
Although bankruptcy questions were raised in the Talmud over 1000 years ago, it was only 20 years ago when a "biblical question" resulted in a renaissance of interest in these fascinating problems.
Joe Malkevitch
York College (CUNY)
malkevitch at york.cuny.edu 
References
Arin, J. and E. Iñarra, A characterization of the nucleolus for convex games, Games and Economic Behavior, 23 (1998) 12-24.
Armstrong, C. Sharing a fish resource: Bargaining theoretical analysis of an applied allocation rule, Marine Policy, 22 (1998) 119-134.
Aumann, R. and M. Maschler, Game theoretic analysis of a bankruptcy problem from the Talmud, J. of Economic Theory, 36 (1985) 195-213.
Benôit, J., The nucleolus is contested-garmet-consistent: A direct proof, J. of Econ. Theory, 77 (1997) 192-196.
Bergantiños, G. and S. Sanchez, The proportional rule for problems with constraints and claims, Mathematical Social Sciences, 43 (2002) 225-249.
Bergantiños, G. and J. Vidal-Puga, Additive rules in bankruptcy problems and other related problems, Mathematical Social Sciences, 47 (2004) 87-101.
Bird, C., On cost allocation for a spanning tree: a game theoretic approach, Networks, 6 (1976) 335-350.
Chun, Y., The equal-loss principle for bargaining problems, Economics Letters, 26 (1988) 103-106.
Chun, Y., The proportional solution for rights problems, Mathematical Social Sciences, 15 (1988) 231-246.
Chun, Y., A new axiomatization of the Shapley value, Games and Economic Behavior, 1 (1989) 119-130.
Chun, Y., A noncooperative justification for egalitarian surplus sharing, Mathematical Social Sciences, 17 (1989) 245-261.
Chun, Y. The equivalence of axioms for bankruptcy problems, International J. of Game Theory, 28 (1999) 510-520.
Chun, Y. and W. Thomson, Bargaining problems with claims, Mathematical Social Sciences, 24 (1992) 19-33.
Curiel, L. and M. Maschler, S. Tijs, Bankruptcy games, Z. Op. Res., 31 (1988) A143-A159.
Dagan, N., New characterizations of old bankruptcy, Social Choice and Welfare, 13 (1996) 51-59.
Dagan, N., A note on Thomson's characterizations of the uniform rule, J. of Economic Theory, 69 (1996) 255-261.
Dagan, N. and O. Voij, The bankruptcy problem: a cooperative bargaining approach, Mathematical Social Sciences, 26 (1993) 287-297.
Dagan, N. and O. Volij, Bilateral comparisons and consistent fair diviion rules in the context of bankruptcy problems, International J. of Game Theory, 26 (1997) 11-25.
Dagan, N. and R. Serrano, O. Volij, A noncooperative view of consistent bankruptcy rules, Games and Economic Behavior, 18 (1997) 55-72.
Dagan, N. and R. Serrano, O. Volij, Feasible implementation of taxation methods, Review of Economic Design, 4 (1999) 57-72.
de Frutos, M., Coalitional manipulation in a bankruptcy problem, Review of Economic Design, 4 (1999) 255-272.
Driessen, T., Tree enterprises and bankruptcy ventures: A game theoretic similarity due to a graph theoretic proof, Discrete Applied Math., 79 (1997) 105-117.
Epstein, I., (ed.), The Babylonian Talmud, Soncino, London, 1935.
Herrero, C. and A. Villar, The three musketeers: four classical solutions to bankruptcy problems, Mathematical Social Sciences, 42 (2001) 307-328.
Littlechild, S. A simple expression for the nucleolus in a special case, International J. of Game Theory, 3 (1974) 21-29.
Littlechild, S. and G. Owen, Aircraft landing fees: a game theory approach, Bell Journal of Economics 8 (1977) 186-204.
Moreno-Ternero, J. and A. Villar, The Talmud rule and the securement of agent's rewards, Mathematical Social Sciences, 47 (2004) 245-257.
Moulin, H., Equal or proportional division of a surplus, and other methods, International J. of Game Theory, 16 (1987) 161-186.
Moulin, H., Axioms of Cooperative Decision Making, Cambridge U. Press, London, 1988.
Moulin, H., Priority rules and other asymmetric rationing methods, Econometrica, 68 (200) 643-684.
Moulin, H., Axiomatic cost and surplus sharing, Chapter 17, in K. Arrow and A. Sen, K. Suzumura, (eds.), Handbook of Social Choice and Wlefare, Elsevier, Amsterdam, 2002, p. 289-357.
Moulin, H. and S. Shenker, Serial cost sharing, Econometrica, 60 (1992) 1009-1037.
O'Neil, B., A problem of rights arbitration from the Talmud, Mathematical Social Sciences, 2 (1982) 345-371.
Obay, B. and H. Orbay, Talmudic division as a cartel rule, J. of Econ. and Business, 55 (2003) 167-175.
Schmeidler, D., The nucleolus of a characteristic function game, SIAM J. of Applied Math., 17 (1969) 1163-1170.
Sen, A., On Economic Inequality, Oxford U. Press, Oxford, 1973.
Thomson, W., The fair division of a fixed supply among a growing population, Math. Oper. Res., 8 (1983) 319-326.
Thomson, W., Consistent solutions to the fair division problem when preferences are single-peaked, J. of Economic Theory, 63 (1994) 219-245.
Thomson, W., Cooperative Models of Bargaining, in Handbook of Game Theory, Chapter 35, Volume 2, R. Aumann, S. Hart, (eds.), North-Holland, Amsterdam, 1995.
Thomson, W., Axiomatic and game-theoretic analyses of bankruptcy and taxation problems: A survey. Mathematical Social Sciences, 45 (2003) 249–297.
Thomson, W. and T. Hokari,, Bankruptcy and weighted generalizations of the the Talmud rule, Economic Theory, 21 (2003) 241-261.
Yaari, M. E. and M. Bar-Hillel, On dividing justly, Social Choice and Welfare, 1 (1984) 1–24.
Yeh, C., Sustainability, exemption, and the constrained equal awards rule, Mathematical Social Sciences, 47 (2004) 103-110.
Young, H., Monotonic solutions of cooperative games, International J. of Game Theory, 14 (1985) 65-72.
Young, H., Distributive justice in taxation, Journal of Economic Theory, 43 (1988) 321–335.
Young, H., Equity in Theory and Practice, Princeton U. Press, Princeton, 1994.
Zhao, J., Dual bargaining and the Talmud bankruptcy problem, 2000 (preprint).
Those who can access JSTOR can find some of the papers mentioned above there. For those with access, the American Mathematical Society's MathSciNet can be used to get additional bibliographic information and reviews of some these materials. Some of the items above can be accessed via the ACM Portal, which also provides bibliographic services.