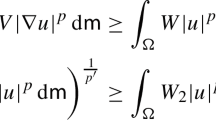Abstract
We establish necessary and sufficient conditions for a domain \({\Omega \subset \mathbb{R}^n}\) to admit the (p, β)-Hardy inequality \({\int_{\Omega} |u|^p d_{\Omega}^{\beta-p} \leq C \int_{\Omega} |\nabla u|^p d_{\Omega}^\beta}\) , where d(x) = dist(x, ∂Ω) and \({u \in C_0^\infty(\Omega)}\) . Our necessary conditions show that a certain dichotomy holds, even locally, for the dimension of the complement Ωc when Ω admits a Hardy inequality, whereas our sufficient conditions can be applied in numerous situations where at least a part of the boundary ∂Ω is “thin”, contrary to previously known conditions where ∂Ω or Ωc was always assumed to be “thick” in a uniform way. There is also a nice interplay between these different conditions that we try to point out by giving various examples.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
Aikawa H.: Quasiadditivity of Riesz capacity. Math. Scand. 69(1), 15–30 (1991)
Aikawa H., Essén M.: ‘Potential theory—selected topics’, lecture notes in mathematics, 1633. Springer, Berlin (1996)
Ancona A.: On strong barriers and an inequality of Hardy for domains in \({\mathbb{R}^n}\) . J. Lond. Math. Soc. (2) 34(2), 274–290 (1986)
Falconer K.: Fractal Geometry. Mathematical foundations and applications. John Wiley & Sons, Ltd., Chichester (1990)
Hajłasz P.: Pointwise Hardy inequalities. Proc. Am. Math. Soc. 127(2), 417–423 (1999)
Hardy G.H., Littlewood J.E., Pólya G.: Inequalities, 2nd edn. Cambridge University Press, Cambridge (1952)
John F.: Rotation and strain. Comm. Pure Appl. Math. 14, 391–413 (1961)
Kinnunen J., Martio O.: Hardy’s inequalities for Sobolev functions. Math. Res. Lett. 4(4), 489–500 (1997)
Koskela P.: The degree of regularity of a quasiconformal mapping. Proc. Am. Math. Soc. 122(3), 769–772 (1994)
Koskela, P., Lehrbäck, J.: ‘Weighted pointwise Hardy inequalities’, preprint (2007)
Koskela P., Zhong X.: Hardy’s inequality and the boundary size. Proc. Am. Math. Soc. 131(4), 1151–1158 (2003)
Lehrbäck J.: Pointwise Hardy inequalities and uniformly fat sets. Proc. Am. Math. Soc. 136(6), 2193–2200 (2008)
Lehrbäck J.: Self-improving properties of weighted Hardy inequalities. Adv. Calc. Var. 1(2), 193–203 (2008)
Lehrbäck J.: Necessary conditions for weighted Hardy inequalities, preprint (2008)
Lewis J.L.: Uniformly fat sets. Trans. Am. Math. Soc. 308(1), 177–196 (1988)
Martio O., Vuorinen M.: Whitney cubes, p-capacity, and Minkowski content. Expo. Math. 5(1), 17–40 (1987)
Mattila P.: Geometry of sets and measures in Euclidean spaces, Cambridge studies in advanced mathematics, vol. 44. Cambridge Univ Press, Cambridge (1995)
Maz’ja V.G.: Sobolev spaces. Springer, Berlin (1985)
Nečas J.: Sur une méthode pour résoudre les équations aux dérivées partielles du type elliptique, voisine de la variationnelle. Ann. Scuola Norm. Sup. Pisa (3) 16, 305–326 (1962)
Smith W., Stegenga D.A.: Hölder domains and Poincaré domains. Trans. Am. Math. Soc. 319, 67–100 (1990)
Stein E.M.: Singular integrals and differentiability properties of functions, Princeton Mathematical Series, No. 30. Princeton University Press, Princeton (1970)
Väisälä J.: Exhaustions of John domains. Ann. Acad. Sci. Fenn. Ser. A I Math. 19(1), 47–57 (1994)
Wannebo A.: Hardy inequalities. Proc. Am. Math. Soc. 109, 85–95 (1990)
Wannebo, A.: Hardy and Hardy PDO type inequalities in domains part I, arXiv:math/ 0401253v1 (http://arxiv.org/abs/math/0401253) (2004)
Author information
Authors and Affiliations
Corresponding author
Additional information
The author was supported in part by the Academy of Finland.
Rights and permissions
About this article
Cite this article
Lehrbäck, J. Weighted Hardy inequalities and the size of the boundary. manuscripta math. 127, 249–273 (2008). https://doi.org/10.1007/s00229-008-0208-5
Received:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00229-008-0208-5