Skip to main content
SpringerLink
Log in

Codimension 3 Nontwisted Double Homoclinic Loops Bifurcations with Resonant Eigenvalues

  • Published:
Journal of Dynamics and Differential Equations Aims and scope Submit manuscript

Abstract

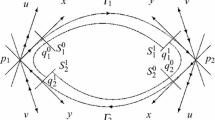
This paper presents the nontwisted double-homoclinic-loop bifurcations with resonant eigenvalues in four dimensional vector fields. The Poincaré map is established to solve various problems in double-homoclinic-loop bifurcations with codimension 3. Bifurcation diagrams and bifurcation curves are given.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Belhaq M., Lakrad F.: Prediction of homoclinic bifurcation: the elliptic averaging method. Chaos Solitons Fractals 11(14), 2251–2258 (2000)

    Article  MATH  MathSciNet  Google Scholar 

  2. Belykh V.N., Bykov V.V.: Bifurcations for heteroclinic orbits of a periodic motion and a saddle-focus and dynamical chaos. Chaos Solitons Fractals 9(2), 1–18 (1998)

    Article  MATH  MathSciNet  Google Scholar 

  3. Chow S.N., Deng B., Fiedler B.: Homoclinic bifurcation at resonant eigenvalues. J. Dyn. Syst. Diff. Eqs. 2, 177–244 (1990)

    Article  MATH  MathSciNet  Google Scholar 

  4. Foroni I., Laura G.: Homoclinic bifurcations in heterogeneous market models. Chaos Solitons Fractals 15(4), 743–760 (2003)

    Article  MATH  MathSciNet  Google Scholar 

  5. Gruendler J.: Homoclinic solutions for autonomous dynamical systems in arbitrary dimension. SIAM J. Math. Anal. 23, 702–721 (1992)

    Article  MATH  MathSciNet  Google Scholar 

  6. Gruendler J.: Homoclinic solutions for autonomous ordinary differential equations with nonautonomous perturbations. J. Diff. Equs. 122, 1–26 (1995)

    Article  MATH  MathSciNet  Google Scholar 

  7. Han M.A., Bi P., Xiao D.M.: Bifurcation of limit cycles and separatrix loops in singular Lienard systems. Chaos Solitons Fractals 20(3), 529–546 (2004)

    Article  MATH  MathSciNet  Google Scholar 

  8. Han M.A., Chen J.: On the number of limit cycles in double homoclinic bifurcations. Sci. China Ser. A 43(9), 914–928 (2000)

    Article  MATH  MathSciNet  Google Scholar 

  9. Homburg A.J., Krauskopf B.: Resonant homoclinic flip bifurcations. J. Dyn. Differ. Equ. 12, 807–850 (2000)

    Article  MATH  MathSciNet  Google Scholar 

  10. Homburg A.J., Knobloch J.: Multiple homoclinic orbits in conservative and reversible systems. Trans. Amer. Math. Soc. 358(4), 1715–1740 (2006)

    Article  MATH  MathSciNet  Google Scholar 

  11. Jin Y.L., Zhu D.M.: Bifurcation of rough heteroclinic loop with two saddle points. Sci. China Ser. A 46, 459–468 (2003)

    MathSciNet  Google Scholar 

  12. Kisaka M., Kokubu H., Oka H.: Bifurcations to n-homoclinic orbits and n-periodic orbits in vector fields. J. Dyn. Differ. Equ. 5, 305–357 (1993)

    Article  MATH  MathSciNet  Google Scholar 

  13. Kokubu H., Komuru M., Oka H.: Multiple homoclinic bifurcations from orbit flip I. Successive homoclinic doublings. Int. J. Bifurcation Chaos 6, 833–850 (1996)

    Article  MATH  Google Scholar 

  14. Liu Z.H., Zhu W.Q.: Homoclinic bifurcation and chaos in simple pendulum under bounded noise excitation. Chaos Solitons Fractals 20(3), 593–607 (2004)

    Article  MATH  Google Scholar 

  15. Lau Y.T.: Global aspects of homoclinic bifurcations of three-dimensional saddles. Chaos Solitons Fractals 3(3), 369–382 (1993)

    Article  MATH  MathSciNet  Google Scholar 

  16. Morales C.A., Pacifico M.J., San Martin B.: Contracting Lorenz attractors through resonant double homoclinic loops. SIAM J. Math. Anal. 8(1), 309–332 (2006)

    Article  MathSciNet  Google Scholar 

  17. Morales C.A., Pacifico M.J., San Martin B.: Expanding Lorenz attractors through resonant double homoclinic loops. SIAM J. Math. Anal. 36(6), 1836–1861 (2005)

    Article  MATH  MathSciNet  Google Scholar 

  18. Oldeman B.E., Krauskopf B., Champneys A.R.: Numerical unfoldings of codimension-three resonant homoclinic flip bifurcations. Nonlinearity 14, 597–621 (2001)

    Article  MATH  MathSciNet  Google Scholar 

  19. Sandstede B.: Constructing dynamical systems having homoclinic bifurcation points of codimension two. J. Dyn. Differ. Equ. 9, 269–288 (1997)

    Article  MATH  MathSciNet  Google Scholar 

  20. Shui S.L., Zhu D.M.: Codimension 3 nonresonant bifurcations of homoclinic orbits with two inclination flips. Sci. China Ser. A 48, 248–260 (2005)

    Article  MATH  MathSciNet  Google Scholar 

  21. Ragazzo C.G.: On the stability of double homoclinic loops. Commun. Math. Phys. 184, 251–272 (1997)

    Article  MATH  Google Scholar 

  22. Tian Q.P., Zhu D.M.: Bifurcation of nontwisted heteroclinic loop. Sci. China Ser. A 43, 818–828 (2000)

    Article  MATH  MathSciNet  Google Scholar 

  23. Wiggins S.: Global Bifurcations and Chaos-Analytical Methods. Springer-Verlag, New York (1988)

    MATH  Google Scholar 

  24. Zhang T.S., Zhu D.M.: Codimension 3 homoclinic bifurcation of orbit flip with resonant eigenvalues corresponding to the tangent directions. Int. J. Bifurcation Chaos 14, 4161–4175 (2004)

    Article  MATH  MathSciNet  Google Scholar 

  25. Zhu D.M., Xia Z.H.: Bifurcations of heteroclinic loops. Sci. China Ser. A 41, 837–848 (1998)

    Article  MATH  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics, East China Normal University, Shanghai, 200062, P.R. China

    Weipeng Zhang, Deming Zhu & Dan Liu

  2. School of Mathematics and Statistics, Northeast Normal University, Changchun, Jilin, 130024, P.R. China

    Weipeng Zhang

Authors
  1. Weipeng Zhang
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Deming Zhu
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Dan Liu
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Deming Zhu.

Additional information

Dedicated to Professor Zhifen Zhang on the occasion of her 80th birthday

Rights and permissions

Reprints and permissions

About this article

Cite this article

Zhang, W., Zhu, D. & Liu, D. Codimension 3 Nontwisted Double Homoclinic Loops Bifurcations with Resonant Eigenvalues. J Dyn Diff Equat 20, 893–908 (2008). https://doi.org/10.1007/s10884-008-9105-6

Download citation

  • Received:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10884-008-9105-6

Keywords

Search

Navigation