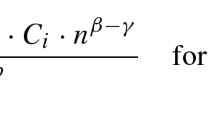Skip to main content
SpringerLink
Log in

Gibbs Distributions for Random Partitions Generated by a Fragmentation Process

  • Published:
Journal of Statistical Physics Aims and scope Submit manuscript

Abstract

In this paper we study random partitions of {1,…,n} where every cluster of size j can be in any of w j possible internal states. The Gibbs (n,k,w) distribution is obtained by sampling uniformly among such partitions with k clusters. We provide conditions on the weight sequence w allowing construction of a partition valued random process where at step k the state has the Gibbs (n,k,w) distribution, so the partition is subject to irreversible fragmentation as time evolves. For a particular one-parameter family of weight sequences w j , the time-reversed process is the discrete Marcus–Lushnikov coalescent process with affine collision rate K i,j = a+b(i+j) for some real numbers a and b. Under further restrictions on a and b, the fragmentation process can be realized by conditioning a Galton–Watson tree with suitable offspring distribution to have n nodes, and cutting the edges of this tree by random sampling of edges without replacement, to partition the tree into a collection of subtrees. Suitable offspring distributions include the binomial, negative binomial and Poisson distributions.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. D. J. Aldous, Deterministic and stochastic models for coalescence (aggregation and coagulation): A review of the mean-field theory for probabilists. Bernoulli 5:3–48 (1999).

    Article  MATH  MathSciNet  Google Scholar 

  2. R. Arratia, A. Barbour and S. Tavaré, Logarithmic combinatorial structures: A probabilistic approach. Eur. Math. Soc. Monogr. 1 (2003).

  3. A. Barbour and B. Granovsky, Random combinatorial structures: The convergent case. J. Comb. Theor., Ser. A 109(2):203–220 (2005).

    Article  MATH  MathSciNet  Google Scholar 

  4. M. H. Bayewitz, J. Yerushalmi, S. Katz and R. Shinnar, The extent of correlations in a stochastic coalescence process. J. Atmos. Sci. 31:1604–1614 (1974).

    Article  ADS  Google Scholar 

  5. J. Berestycki, Exchangeable fragmentation-coalescence processes and their equilibrium distribution. Electr. J. Prob. 9:770–824 (2004).

    MathSciNet  Google Scholar 

  6. J. Berestycki, N. Berestycki and J. Schweinsberg, Small-time behavior of Beta-coalescents. To appear in Ann. Inst. H. Poincar (B). Probabilités et Statistiques. Preprint, math.PR/0601032 (2006).

  7. J. Berestycki, N. Berestycki and J. Schweinsberg, Beta-coalescents and continuous stable random trees. To appear in Ann. Probab. Preprint, math.PR/0602113 (2006).

  8. N. Berestycki, The hyperbolic geometry of random transpositions. Ann. Probab. 34(2):429–467 (2006).

    Article  MATH  MathSciNet  Google Scholar 

  9. L. Comtet, Advanced Combinatorics. D. Reidel Pub. Co., Boston (1974). (translated from French).

    MATH  Google Scholar 

  10. P. C. Consul and F. Famoye, Lagrangian Probability Distributions (Birkhäuser Boston Inc., Boston, MA, 2006).

    MATH  Google Scholar 

  11. P. C. Consul and L. R. Shenton, Use of Lagrange expansion for generating discrete generalized probability distributions, SIAM J. Appl. Math. 23:239–248 (1972).

    Article  MATH  MathSciNet  Google Scholar 

  12. L. Devroye, The branching process method in the Lagrange random variate generation. Commun. Stat.–-Simulation 21:1–14 (1992).

    MATH  MathSciNet  Google Scholar 

  13. A. Di Bucchianico, Probabilistic and Analytical Aspects of the Umbral Calculus, CWI Tract, vol. 119. (Stichting Mathematisch Centrum Centrum voor Wiskunde en Informatica, Amsterdam, 1997).

    Google Scholar 

  14. R. Dong, A. Gnedin and J. Pitman, Exchangeable partitions derived from Markovian coalescents. Preprint, math.PR/0603745.

  15. R. Durrett, B. L. Granovsky and S. Gueron, The equilibrium behavior of reversible coagulation-fragmentation processes. J. Theoret. Probab. 12(2):447–474 (1999).

    Article  MATH  MathSciNet  Google Scholar 

  16. R. Durrett and J. Schweinsberg, Power laws for family sizes in a duplication model. Ann. Probab. 33(6):2094–2126 (2005).

    Article  MATH  MathSciNet  Google Scholar 

  17. M. Erlihson and B. Granovsky, Reversible coagulation-fragmentation processes and random combinatorial structures: asymptotics for the number of groups. Rand. Struct. Algor. 25:227–245 (2004).

    Article  MATH  MathSciNet  Google Scholar 

  18. P. Erdös, R. K. Guy and J. W. Moon, On refining partitions, J. London Math. Soc., Ser. II 9:565–570 (1975).

    Article  MATH  Google Scholar 

  19. W. J. Ewens, The sampling theory of selectively neutral alleles, Theor. Popul. Biol. 3:87–112 (1972).

    Article  MathSciNet  Google Scholar 

  20. P. J. Flory. Principles of Polymer Chemistry. (Cornell University Press, Ithaca, London, 1953).

    Google Scholar 

  21. B. Fristedt, The structure of partitions of large integers. Trans. Am. Math. Soc. 337:703–735 (1993).

    Article  MATH  MathSciNet  Google Scholar 

  22. A. Gnedin and J. Pitman, Exchangeable Gibbs partitions and Stirling triangles, Zapiski St. Petersb. Dept. Math. Inst. 325:82-103 (2005).

    Google Scholar 

  23. A. Gnedin and J. Pitman, Poisson representation of a Ewens fragmentation process. Preprint, arXiv:math.PR/0608307.

  24. W. M. Y. Goh and E. Schmutz, Random set partitions. SIAM J. Discrete Math. 7:419–436 (1994).

    Article  MATH  MathSciNet  Google Scholar 

  25. H. W. Gould, A series of transformations leading to convolution identities. Duke Math. J. 28:193–202 (1961).

    Article  MathSciNet  MATH  Google Scholar 

  26. B. Haas, G. Miermont, J. Pitman and M. Winkel, Continuum tree asymptotics of discrete fragmentations and applications to phylogenetic models. Preprint, math.PR/0604350.

  27. L. H. Harper, The morphology of partially ordered sets. J. Comb. Theor. Ser. A 17:44–58 (1974).

    Article  MATH  MathSciNet  Google Scholar 

  28. E. M. Hendriks, J. L. Spouge, M. Eibl and M. Shreckenberg, Exact solutions for random coagulation processes. Z. Phys. B—Condensed Matter 58:219–227 (1985).

    Article  ADS  Google Scholar 

  29. L. Holst, On the lengths of the pieces of a stick broken at random. J. Appl. Probab. 17:623–634 (1980).

    Article  MATH  MathSciNet  Google Scholar 

  30. L. Holst, On numbers related to objects of unlike partitions and occupancy problems. Eur. J. Combin. 2(3):231–237 (1981).

    MATH  MathSciNet  Google Scholar 

  31. S. Janson, Conditioned Galton—Watson trees do not grow. Preprint, math.PR/0604141.

  32. T. Kamae, U. Krengel and G.L. O'Brien, Stochastic inequalities on partially ordered spaces. Ann. Probab. 5:899–912 (1977).

    MATH  MathSciNet  Google Scholar 

  33. F. Kelly. Reversibility in Stochastic Networks. (Wiley, 1979).

  34. J. F. C. Kingman, The coalescent. Stochastic Processes Their Appl. 13:235–248 (1982).

    Article  MATH  MathSciNet  Google Scholar 

  35. J. F. C. Kingman, On the genealogy of large populations. In Essays in Stastical Science, J. Gani and E. J. Hannan, eds. (Applied Probability Trust, Sheffield). J. Appl. Prob. Spec. 19A:27–43 (1982).

    Google Scholar 

  36. V. F. Kolchin. Random Graphs. (Cambridge University Press, Cambridge, 1999).

    MATH  Google Scholar 

  37. V. F. Kolchin. Random Mappings. Translation series in Mathematics and Engineering. Optimization Software Inc. Publications Division, New York, 1986. MR88:a60022.

    Google Scholar 

  38. D. Knuth, Convolution polynomials. Mathematica Journal 2(4):67–78 (1992). Reprinted as Chapter 41 of Selected Papers of Discrete Mathematics (Stanford, California, Center for the Study of Language and Information). Available from the arXiv server as math.CA/9207221.

    MathSciNet  Google Scholar 

  39. A. A. Lushnikov, Coagulation in finite systems. J. Colloid and Interface Sci. 65:276–285 (1978).

    Article  Google Scholar 

  40. A. H. Marcus, Stochastic coalescence. Technometrics 10:133–143 (1968).

    Article  MathSciNet  Google Scholar 

  41. M. Möhle, On sampling distributions for coalescent processes with simultaneous multiple collisions. Bernoulli 12(1):35–53 (2006).

    MATH  MathSciNet  Google Scholar 

  42. J. Pitman, Enumerations of trees and forests related to branching processes and random walks. Microsurveys in Discrete Probability, D. Aldous and J. Propp, eds. DIMACS Ser. Discrete Math. Theoret. Comp. Sci no. 41, 163–180. Am. Math. Soc. Providence, RI (1998).

    Google Scholar 

  43. J. Pitman, Coalescent random forests. J. Combin. Theor. A. 85:165–193 (1999).

    Article  MATH  MathSciNet  Google Scholar 

  44. J. Pitman, Combinatorial stochastic processes. Lecture notes in mathematics, Ecole d'Eté de probabilités de Saint-Flour XXXII-2002. Vol. 1875, Springer, 2006.

  45. A. Rényi, Probabilistic methods in combinatorial mathematics. In R. C. Bose and T. A. Dowlilng, eds. Combinatorial Mathematics and its Applications. (University of North Carolina Press, Chapel Hill, 1969, pp. 1–13).

    Google Scholar 

  46. R. P. Stanley, Enumerative Combinatorics. Vol. 2, volume 62 of Cambridge Studies in Advanced Mathematics. (Cambridge University Press, Cambridge, 1999).

    Google Scholar 

  47. A. M. Vershik, Statistical mechanics of combinatorial partitions and their limit shapes. Funct. Anal. Appl. 30:90–105 (1996). (translation from Russian).

    Article  MATH  MathSciNet  Google Scholar 

  48. P. Whittle, The equilibrium statistics of a clustering process in uncondensed phase. Proc. Roy. Lond. Soc. A 285:501–519 (1965).

    MATH  ADS  Google Scholar 

  49. P. Whittle, Statistical processes of aggregation and polymerisation. Proc. Camb. Phil. Soc. 61:475–495 (1965).

    Article  MathSciNet  Google Scholar 

  50. P. Whittle. Systems in Stochastic Equilibrium. (Wiley, 1986).

Download references

Author information

Authors and Affiliations

  1. University of British Columbia, Vancouver, B.C., Canada, V6T 1Z2

    Nathanaël Berestycki & Jim Pitman

Authors
  1. Nathanaël Berestycki
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Jim Pitman
    View author publications

    You can also search for this author in PubMed Google Scholar

Additional information

Research supported in part by N.S.F. Grant DMS-0405779.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Berestycki, N., Pitman, J. Gibbs Distributions for Random Partitions Generated by a Fragmentation Process. J Stat Phys 127, 381–418 (2007). https://doi.org/10.1007/s10955-006-9261-1

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10955-006-9261-1

Keywords

Search

Navigation