Skip to main content
SpringerLink
Log in

Percolation Properties of the Non-ideal Gas

  • Published:
Journal of Statistical Physics Aims and scope Submit manuscript

Abstract

We estimate locations of the regions of the percolation and of the non-percolation in the plane (λ,β): the Poisson rate–the inverse temperature, for interacting particle systems in finite dimension Euclidean spaces. Our results about the percolation and about the non-percolation are obtained under different assumptions. The intersection of two groups of the assumptions reduces the results to two dimension Euclidean space, ℝ2, and to a potential function of the interactions having a hard core.

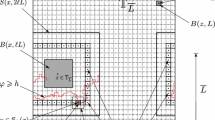
The technics for the percolation proof is based on a contour method which is applied to a discretization of the Euclidean space. The technics for the non-percolation proof is based on the coupling of the Gibbs field with a branching process.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Fernández, R.: Contour ensembles and the description of Gibbsian probability distributions at low temperature. In: Notes for a Minicours Given at the 21 Colôquio Brasileiro de Matemática. IMPA, Rio de Janeiro (1997)

    Google Scholar 

  2. Kac, M., Uhlenbeck, G., Hemmer, P.C.: On the Van der Waals theory of vapor-liquid equilibrium. J. Math. Phys. 5, 60–74 (1964)

    Article  MathSciNet  ADS  Google Scholar 

  3. Lebowitz, J.L., Mazel, A., Presutti, E.: Liquid-vapor phase transitions for systems with finite range interactions. J. Stat. Phys. 94, 955–1027 (1999)

    Article  MathSciNet  MATH  Google Scholar 

  4. Malyshev, V.A., Minlos, R.A.: Gibbs Random Fields, Cluster Expansions. Kluwer Acad., Dordrecht (1991)

    MATH  Google Scholar 

  5. Meester, R., Roy, R.: Continuum Percolation. Cambridge Tracts in Mathematics, vol. 119. Cambridge University Press, Cambridge (1996)

    MATH  Google Scholar 

  6. Pechersky, E., Zhukov, Yu.: Uniqueness of Gibbs state for non-ideal gas in ℝd: the case of pair potentials. J. Stat. Phys. 97(1/2), 145–172 (1999)

    Article  MathSciNet  MATH  Google Scholar 

  7. Ruelle, D.: Superstable interactions in classical statistical mechanics. Commun. Math. Phys. 18, 127–159 (1970)

    Article  MathSciNet  ADS  MATH  Google Scholar 

  8. Ferrari, P., Pechersky, E., Sisko, V., Yambartsev, A.: Gibbs random graphs (in preparation)

  9. Sinai, Ya.G.: Theory of Phase Transitions. Rigorous Results. Pergamon, Oxford (1982)

    MATH  Google Scholar 

  10. Mürmann, M.G.: Equilibrium distribution of physical clusters. Commun. Math. Phys. 45, 233–246 (1975)

    Article  ADS  MATH  Google Scholar 

  11. Zessin, H.: A theorem of Michael Mürmann revisited. J. Contemp. Math. Anal. 43(1), 68–80 (2008)

    Google Scholar 

  12. Dobrushin, R.L.: Gibbsian random fields for particles without hard core. Theor. Math. Phys. 4(1), 705–719 (1970)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. IITP, 19, Bolshoj Karetny per., Moscow, Russia

    E. Pechersky

  2. IME-USP, Rua do Matão, 1010, 05508-090, São Paulo, Brazil

    A. Yambartsev

Authors
  1. E. Pechersky
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. A. Yambartsev
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to E. Pechersky.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Pechersky, E., Yambartsev, A. Percolation Properties of the Non-ideal Gas. J Stat Phys 137, 501–520 (2009). https://doi.org/10.1007/s10955-009-9856-4

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10955-009-9856-4

Search

Navigation