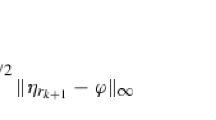Skip to main content
SpringerLink
Log in

Hausdorff and Packing Measures of the Level Sets of Iterated Brownian Motion

  • Published:
Journal of Theoretical Probability Aims and scope Submit manuscript

Abstract

Burdzy and Khoshnevisan(9) have shown that the Hausdorff dimension of the level sets of an iterated Brownian motion (IBM) is equal to 3/4. In this paper, the exact Hausdorff measure function and the packing measure of the levels set of IBM are given. Our approach relies on some accurate analysis on the local asymptotic of local times.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

REFERENCES

  1. Arcones, M. A. (1995). On the law of the iterated logarithm for Gaussian processes. J. Theoret. Prob. 8, 877–903.

    Google Scholar 

  2. Barlow, M. T., Perkins, E. A., and Taylor, S. J. (1986). Two uniform intrinsic constructions for the local time of a class of Lévy processes. Illinois J. Math. 30, 19–65.

    Google Scholar 

  3. Benachour, S., Roynette, B., and Vallois, P. (1996). Une approche probabiliste de l'équation u t =-1/8(∂2 u/∂x 4). Preprint.

  4. Bertoin, J. (1996). Iterated Brownian motion and stable (1/4) subordinator. Statist. Prob. Letters 27, 111–114.

    Google Scholar 

  5. Bertoin, J., and Shi, Z. (1996). Hirsch's integral test for the iterated Brownian motion. In J. Azéma, M. Émery, and M. Yor (eds.), Sém. Probab. XXX, Lect. Notes in Math., Springer, Berlin, 1626, 361–368.

    Google Scholar 

  6. Biane, Ph., and Yor, M. (1988). Sur la loi des temps locaux Browniens pris en un temps exponentiel. Sém. Probab. XXII, Lect. Notes in Math., Springer, Berlin, 1321, 454–466.

    Google Scholar 

  7. Burdzy, K. (1993). Some path properties of iterated Brownian motion. In E. Çinlar, K. L. Chung, and M. Sharpe (eds.), Seminar on Stochastic Processes, Birkhäuser, Boston, pp. 67–87.

    Google Scholar 

  8. Burdzy, K. (1994). Variation of iterated Brownian motion. In D. A. Dawson (ed.), Measure-Values Processes, Stochastic Partial Equations and Interacting Systems, CRM Proc. Lect. Notes 5, 35–53.

  9. Burdzy, K., and Khoshnevisan, D. (1995). The levels sets of iterated Brownian motion. In J. Azéma, M. Émery, P. A. Meyer, and M. Yor (eds.), Sém. Probab. XXIX, Lect. Notes in Math., Springer, Berlin, 1613, 231–236.

    Google Scholar 

  10. Burdzy, K., and Khoshnevisan, D. (1998). Brownian motion in a Brownian crack. Ann. Appl. Prob. 8, 708–748.

    Google Scholar 

  11. Chung, K. L. (1948). On the maximum partial sums of sequences of independent random variables. Trans. Amer. Math. Soc. 64, 205–233.

    Google Scholar 

  12. Csáki, E., Csörgő, M., Földes, A., and Révész, P. (1995). Global Strassen type theorems for iterated Brownian motion. Stoch. Proc. Appl. 59, 321–341.

    Google Scholar 

  13. Csáki, E., Csörgő, M., Földes, A., and Révész, P. (1996). The local time of iterated Brownian motion. J. Theoret. Prob. 9, 717–743.

    Google Scholar 

  14. Csáki, E., Csörgő, M., Földes, A., and Révész, P. (1997). On the occupation time of an iterated process having no local time. Stoch. Proc. Appl. 70, 199–217.

    Google Scholar 

  15. Csáki, E., Földes, A., and Révész, P. (1997). Strassen-type theorems for a class of iterated processes. Trans. Amer. Math. Soc. 349, 1153–1167.

    Google Scholar 

  16. Csörgő, M., and Révész, P. (1981). Strong Approximations in Probability and Statistics, Academic Press, New York.

    Google Scholar 

  17. Eisenbaum, N., and Shi, Z. (1999). Uniform oscillations of the local time of iterated Brownian motion. Bernoulli (to appear).

  18. Fristedt, B. E., and Taylor, S. J. (1992). The packing measure of a general subordinator. Prob. Theor. Rel. Fields 92, 493–510.

    Google Scholar 

  19. Funaki, T. (1979). A probabilistic construction of the solution of some higher order parabolic differential equations. Proc. Japan Acad. 55, 176–179.

    Google Scholar 

  20. Hu, Y., Pierre-Loti-Viaud, D., and Shi, Z. (1995). Laws of the iterated logarithm for iterated Wiener processes. J. Theor. Prob. 8, 303–319.

    Google Scholar 

  21. Hu, Y., and Shi, Z. (1995). The Csörgő-Révész modulus of non-differentiability of iterated Brownian motion. Stoch. Proc. Appl. 58, 267–279.

    Google Scholar 

  22. Hochberg, K. J., and Orsingher, E. (1996). Composition of stochastic processes governed by higher-order parabolic and hyperbolic equations. J. Theor. Probab. 9, 511–532.

    Google Scholar 

  23. Khoshnevisan, D., and Lewis, T. M. (1996). Chung's law of the iterated logarithm for the iterated Brownian motion. Ann. Inst. Henri Poincaré 32, 349–359.

    Google Scholar 

  24. Khoshnevisan, D., and Lewis, T. M. (1996). The modulus of continuity for iterated Brownian motion. J. Theor. Prob. 9, 317–333.

    Google Scholar 

  25. Khoshnevisan, D., and Lewis, T. M. (1999). Stochastic calculus for Brownian motion on a Brownian fracture. Ann. Appl. Prob. (to appear).

  26. Kochen, S. B., and Stone, C. J. (1964). A note on the Borel-Cantelli lemma. Illinois J. Math. 8, 248–251.

    Google Scholar 

  27. Perkins, E. (1980). The exact Hausdorff measure of the level sets of Brownian motion. Z. Wahrsch. verw Geb. 58, 373–388.

    Google Scholar 

  28. Perkins, E. (1981). On the iterated logarithm law for local time. Proc. Amer. Math. Soc. 81, 470–472.

    Google Scholar 

  29. Pruitt, W. E., and Taylor, S. J. (1996). Packing and covering indices for a general Lévy process. Ann. Prob. 24, 971–986.

    Google Scholar 

  30. Revuz, D., and Yor, M. (1994). Continuous Martingales and Brownian Motion, Second Edition, Springer, Berlin.

    Google Scholar 

  31. Shi, Z. (1995). Lower limits of iterated Wiener processes. Statist. Prob. Lett. 23, 259–270.

    Google Scholar 

  32. Shi, Z., and Yor, M. (1997). Integrability and lower limits of the local time of iterated Brownian motion. Studia Scientiarum Math. Hung. 33, 279–298.

    Google Scholar 

  33. Takashima, K. (1989). Sample path properties of ergodic self-similar processes. Osaka J. Math. 26, 159–189.

    Google Scholar 

  34. Taylor, S. J. (1986). The measure theory of random fractals. Math. Proc. Camb. Phi. Soc. 100, 383–425.

    Google Scholar 

  35. Taylor, S. J. (1987). The use of packing measure in the analysis of random sets. Proc. 15th Symp. Stoch. Proc. Appl., Springer, Berlin, Lect. Notes Math. 1203, 214–222.

    Google Scholar 

  36. Taylor, S. J., and Tricot, C. (1985). Packing measure and its evaluation for a Brownian path. Trans. Amer. Math. Soc. 288, 679–699.

    Google Scholar 

  37. Taylor, S. J., and Wendel, J. G. (1966). The exact Hausdorff measure of the zero set of a stable process. Z. Wahrsch. verw Geb. 6, 170–180.

    Google Scholar 

  38. Xiao, Y. (1997). Hölder conditions for the local times and the Hausdorff measure of the level sets of Gaussian random fields. Prob. Theor. Rel. Fields 109, 129–157.

    Google Scholar 

  39. Xiao, Y. (1998). Local times and related properties of multi-dimensional iterated Brownian motion. J. Theor. Prob. 11, 383–408.

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Laboratoire de Probabilités, CNRS UMR 7599, Université Paris VI, Tour 56, 4 Place Jussieu, F-75252, Paris Cedex 05, France

    Yueyun Hu

Authors
  1. Yueyun Hu
    View author publications

    You can also search for this author in PubMed Google Scholar

Rights and permissions

Reprints and permissions

About this article

Cite this article

Hu, Y. Hausdorff and Packing Measures of the Level Sets of Iterated Brownian Motion. Journal of Theoretical Probability 12, 313–346 (1999). https://doi.org/10.1023/A:1021669809625

Download citation

  • Issue Date:

  • DOI: https://doi.org/10.1023/A:1021669809625

Search

Navigation