Abstract
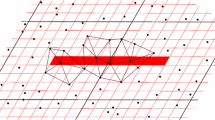
In the present study we establish a phase transition in the nearest-neighbor continuum Potts model. The repulsion between particles of different type acts only on a nearest-neighbor graph, more precisely a subgraph of the Delaunay graph. This work is an adaptation of the Lebowitz and Lieb soft-core continuum Potts model.
Similar content being viewed by others
REFERENCES
A. Baddeley and J. Møller, Nearest-neighbor Markov point processes and random sets, Int. Statist. Rev. 57:89–121 (1989).
E. Bertin, J.-M. Billiot, and R. Drouilhet, Existence of Delaunay pairwise Gibbs point processes with superstable component, J. Statist. Phys. 95:719–744 (1999).
E. Bertin, J.-M. Billiot, and R. Drouilhet, Existence of “nearest-neighbor” Gibbs point models, Adv. Appl. Prob. 31:895–909 (1999).
E. Bertin, J.-M. Billiot, and R. Drouilhet, k-Nearest-neighbor Gibbs point processes, Markov Process. Related Fields 5:219–234 (1999).
E. Bertin, J.-M. Billiot, and R. Drouilhet, Spatial Delaunay Gibbs point processes, Stochastic Models 15:181–199 (1999).
E. Bertin, J.-M. Billiot, and R. Drouilhet, Continuum percolation in the Gabriel graph, Adv. Appl. Prob. 34:689–701 (2002).
A. Bowyer, Computing Dirichlet tessellations, Comput. J. 24:162–166 (1981).
J. Chayes, L. Chayes, and R. Kotecky, The analysis of the Widom-Rowlinson model by stochastic geometric methods, Commun. Math. Phys. 172:551–569 (1995).
R. Connelly, K. Rybnikov, and S. Volkov, Percolation of the loss of tension in an infinite triangular lattice, J. Statist. Phys. 105:143–171 (2001).
G. Döge, K. Mecke, J. Møller, D. Stoyan, and R. Waagepetersen, Grand canonical simulations of hard-disk systems by simulated tempering, Technical Report R-00-2003, Department of Mathematical Sciences, Aalborg University (2000).
H.-O. Georgii, Large deviations and the equivalence of ensembles for Gibbsian particle systems with superstable interaction, Probab. Theory Related Fields 99:171–195 (1994).
H.-O. Georgii and O. Häggström, Phase transition in continuum Potts models, Commun. Math. Phys. 181:507–528 (1996).
H.-O. Georgii and V. Zagrebnov, On the interplay of magnetic and molecular forces in Curie-Weiss ferrofluid models, J. Statist. Phys. 93:79–107 (1998).
C. Geyer, Likelihood inference for spatial point processes, in Stochastic Geometry, Likelihood and Computation, W. S. Kendall, O. E. Barndoff-Nielsen, and M. N. M. van Lieshout, eds. (Chapman & Hall, London, 1999), pp. 141–172.
C. Geyer and J. Møller, Simulation procedures and likelihood inference for spatial point processes, Scand. J. Statist. 21:359–373 (1994).
O. Häggström, Markov random fields and percolation on general graphs, Adv. Appl. Prob. 32:39–66 (2000).
O. Häggström and R. Meester, Nearest neighbor and hard sphere models in continuum percolation, Rand. Struct. Algorithms 9:295–315 (1996).
J. Lebowitz and E. Lieb, Phase transition in a continuum classical system with finite interactions, Phys. Lett. A 39:98–100 (1972).
J. Lebowitz, A. Mazel, and E. Presutti, Liquid vapor phase transition for systems with finite range interactions, J. Statist. Phys. 516:955–1025 (1999).
R. Lyons and Y. Peres, Probability on Trees and Networks (Cambridge University Press, Cambridge, 2002).
S. Mase, J. Møller, D. Stoyan, R. Waagepetersen, and G. Döge, Packing densities and simulated tempering for hard-core gibbs point processes, Ann. Inst. Statist. Math. 53:661–680 (2001).
R. W. J. Meester and R. Roy, Continuum Percolation (Cambridge University Press, Cambridge, 1996).
J. Møller, Extensions of the Swendsen-Wang algorithm for simulating spatial point process, Technical Report 246, Department of Theoretical Statistics, Institute of Mathematics, University of Aarhus (1992).
J. Møller, Lectures on random Voronoi tessellations, in Lecture Notes in Statistics, Vol. 87 (Springer-Verlag, New York, 1994).
C. Preston, Spatial birth and death processes, Bull. Int. Statist. Inst. 46:371–391 (1977).
B. Ripley, Modelling spatial patterns (with discussion), J. R. Statist. Soc. B 39:172–212 (1977).
D. Ruelle, Existence of a phase transition in a continuous classical system, Phys. Rev. Lett. 27:1040–1041 (1971).
A. van Enter, R. Fernández, and A. Sokal, Regularity properties and pathologies of position-space renormalization-group transformations, J. Statist. Phys. 72:879–1152 (1994).
B. Widom and J. Rowlinson, New model for the study of liquid-vapor transitions, J. Chem. Phys. 52:1670–1684 (1970).
Author information
Authors and Affiliations
Rights and permissions
About this article
Cite this article
Bertin, E., Billiot, JM. & Drouilhet, R. Phase Transition in the Nearest-Neighbor Continuum Potts Model. Journal of Statistical Physics 114, 79–100 (2004). https://doi.org/10.1023/B:JOSS.0000003056.84984.8b
Issue Date:
DOI: https://doi.org/10.1023/B:JOSS.0000003056.84984.8b