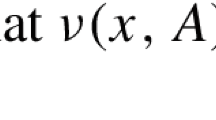Skip to main content
SpringerLink
Log in

On Exit and Ergodicity of the Spectrally One-Sided Lévy Process Reflected at Its Infimum

  • Published:
Journal of Theoretical Probability Aims and scope Submit manuscript

Abstract

Consider a spectrally one-sided Lévy process X and reflect it at its past infimum I. Call this process Y. For spectrally positive X, Avram et al.(2) found an explicit expression for the law of the first time that Y=X−I crosses a finite positive level a. Here we determine the Laplace transform of this crossing time for Y, if X is spectrally negative. Subsequently, we find an expression for the resolvent measure for Y killed upon leaving [0,a]. We determine the exponential decay parameter ϱ for the transition probabilities of Y killed upon leaving [0,a], prove that this killed process is ϱ-positive and specify the ϱ-invariant function and measure. Restricting ourselves to the case where X has absolutely continuous transition probabilities, we also find the quasi-stationary distribution of this killed process. We construct then the process Y confined in [0,a] and prove some properties of this process.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

references

  1. Asmussen, S. (1989). Applied Probability and Queues, Wiley series in probability.

  2. Avram, F., Kyprianou, A. E., and Pistorius, M. R. (2004). Exit problems for spectrally negative Lévy processes and applications to (Canadized) Russian options. Ann. Appl. Probab. (forthcoming).

  3. Bertoin, J. (1991). FrSur la décomposition de la trajectoire d'un processus de Lévy spectralement positif en son infimum. Ann. Inst. H. Poincaré Probab. Statist. 27, 537-547.

    Google Scholar 

  4. Bertoin, J. (1996). On the first exit time of a completely asymmetric stable process from a finite interval. Bull. London Math. Soc. 28, 514-520.

    Google Scholar 

  5. Bertoin, J. (1996). Lévy Processes, Cambridge University Press.

  6. Bertoin, J. (1997). Exponential decay and ergodicity of completely asymmetric Lévy processes in a finite interval. Ann. Appl. Probab. 7, 156-169.

    Google Scholar 

  7. Bingham, N. H. (1975). Fluctuation theory in continuous time. Adv. Appl. Prob. 7, 705-766.

    Google Scholar 

  8. Borovkov, A. A. (1976). Stochastic Processes in Queueing Theory, Springer-Verlag.

  9. Borodin, A. N., and Salminen, P. (1996). Handbook of Brownian Motion—Facts and Formulae, Birkhäuser.

  10. Chaumont, L. (1993). FrSur certains processus de Lévy conditionés a rester positifs. Stochastics Stochastic Rep. 47, 1-20.

    Google Scholar 

  11. Chung, K. L. (1986). Doubly Feller process with multiplicative functional. In Seminar on Stochastic Processes, Birkhäuser, pp. 63-78.

  12. Dellacherie, C., and Meyer, P. A. (1987). FrProbabilités et potentiel (tome 4), Hermann Paris.

  13. Emery, D. J. (1973). Exit problem for a spectrally positive process. Adv. in Appl. Probab. 5, 498-520.

    Google Scholar 

  14. Hawkes, J. (1979). Potential theory of Lévy processes. Proc. London Math. Soc. 38, 335-352.

    Google Scholar 

  15. Jacod, J., and Shiryaev, A. N. (1987). Limit Theorems for Stochastic Processes, Springer-Verlag.

  16. Knight, F. B. (1969). Brownian local times and taboo processes. Trans. Amer. Soc 73, 173-185.

    Google Scholar 

  17. Kyprianou, A. E., and Pistorius, M. R. (2003). Perpetual options and Canadization through fluctuation theory. Ann. Appl. Probab. 13, 1077-1098.

    Google Scholar 

  18. Lambert, A. (2000). Completely asymmetric Lévy processes confined in a finite interval. Ann. Inst. H. Poincaré Prob. Stat. 36, 251-274.

    Google Scholar 

  19. Winkel, M. (2002). Right inverses of non-symmetric Lévy processes. Ann. Probab. 30, 382-415.

    Google Scholar 

  20. Prabhu, N. U. (1997). Insurance, Queues, Dams, Springer-Verlag.

  21. Robbins, H., and Siegmund, D. (1972). On the law of the iterated logarithm for maxima and minima. Proc. Sixth Berkerley Symp. 3, 51-70.

    Google Scholar 

  22. Protter, P. (1990). Stochastic Integration and Differential Equations, Springer-Verlag.

  23. Rogers, L. C. G. (1984). On a new identity for real Lévy processes. Ann. Inst. Henri Poincaré 20, 21-34.

    Google Scholar 

  24. Spitzer, F. (1964). Principles of Random Walks, Van Nostrand, Princeton.

    Google Scholar 

  25. Suprun, V. N. (1976). The ruin problem and the resolvent of a killed independent increment process. Ukrainian Math. J. 28, 39-45.

    Google Scholar 

  26. Takács, L. (1966). Combinatorial Methods in the Theory of Stochastic Processes, Wiley, New York.

    Google Scholar 

  27. Tucker, H. G. (1962). Absolute continuity of infinitely divisible distributions. Pacific J. Math. 12, 1125-1129.

    Google Scholar 

  28. Tuominen, P., and Tweedie, R. (1979). Exponential decay and ergodic properties of general Markov processes. Adv. Appl. Prob. 11, 784-803.

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics, King's College London, Strand, London, WC2R 2LS, United Kingdom

    M. R. Pistorius

Authors
  1. M. R. Pistorius
    View author publications

    You can also search for this author in PubMed Google Scholar

Rights and permissions

Reprints and permissions

About this article

Cite this article

Pistorius, M.R. On Exit and Ergodicity of the Spectrally One-Sided Lévy Process Reflected at Its Infimum. Journal of Theoretical Probability 17, 183–220 (2004). https://doi.org/10.1023/B:JOTP.0000020481.14371.37

Download citation

  • Issue Date:

  • DOI: https://doi.org/10.1023/B:JOTP.0000020481.14371.37

Search

Navigation