Reading the Bakhshali Manuscript
The Bakhshali manuscript is in a very damaged state, but is a valuable mathematical record nonetheless...
 Bill Casselman
Bill Casselman
University of British Columbia, Vancouver, Canada
Email Bill Casselman
Introduction
The Bakhshali manuscript is a mathematical document found in 1881 by a local farmer in the vicinity of the village of Bakhshali, near the city of Peshawar in what was then British India and is now Pakistan. It is written in ink on birch bark, a common medium for manuscripts in northwestern India throughout much of history. In the tough climate of India and neighbouring regions, such things deteriorate rapidly, and it is miraculous that this document has survived.
The Bakhshali manuscript is in a very damaged state, but is a valuable mathematical record nonetheless. It consists now of $70$ pages, but was probably once part of something much longer. Some of the pages we have are themselves broken up into fragments, and large parts are missing. Even the exact order of the pages has been a matter of conjecture, since the state in which it first came under careful examination is not necessarily the original order. The first edition of the manuscript was published by the Government of India in Calcutta in 1927, and its editor was G. R. Kaye. In 1995 a new edition was published, edited by Takao Hayashi as an extension of his PhD thesis at Brown University. He ordered the pages very differently from Kaye, and made a much more thorough translation.
The manuscript was donated to the Bodleian Library at Oxford University early in the twentieth century. Attempts to assess its age have generated much controversy--estimates have ranged, roughly, from 300 C.E. to 1200 C.E. In the summer of 2017 the Bodleian Library applied radio carbon dating techniques to a few fragments of bark taken from it, and announced what it considered indisputable estimates of its age. Unfortunately, their dating seems only to have added to the controversy. I'll say more about this later. One point to keep in mind is that the manuscript is almost certainly a copy of a much earlier one, so that the physical date of the pages we have is not likely to be the date of the original composition. Nor is the scribe who made the copy likely to be the original author.
Some big numbers
As far as content goes, the Bakhshali manuscript does not bring us much that is not found in other works in Indian mathematics. It is even perhaps a bit duller than most of the others that have survived, except in a couple of features. It doesn't seem to have any particular theme, and basically amounts to a motley collection of problems and solutions that are unrelated to each other. The solutions are sometimes laid out in excruciating detail. Mathematically, its claim to fame is that it contains an extraordinarily accurate approximation to the square roots of integers that are not perfect squares. But much of its interest to the world at large is that it might seem to contribute to answering some basic questions. When and where was the decimal system of calculation invented? In particular, who invented "0"? But whether it actually does tell us something new about the history of decimal notation is not clear.
In any case, one point is that the Bakhshali manuscript is full of numbers expressed in decimal notation and even full of decimal calculations, and extremely rich in "0"s. Take a look at my favourite mansucript page.

| |
This is from page 46 in the Bodleian's copy - 46r or 46 recto in the standard jargon (as opposed to the following page on the opposite side, which is 46v or 46 verso).
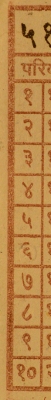
At first this undoubtedly looks just very confusing, since the symbols will be unfamiliar to most of those reading this. But if you look carefully for a while you will see that certain symbols are repeated often. In fact, 10 symbols essentially cover the page. These are the ones in the list below (which I copied from Kaye's book):

That is to say, the page is covered with symbols for the digits 0 - 9. They are the ancestors, in truth, of our modern digits, although tracking the transformations from ancient to modern makes a long story. Also, I have to remind you that "modern" is a matter of place as well as time. Some of these numerals will probably look reasonably familar to anyone acquainted with Indian culture. I include at the right the digits found on a recent bus ticket from Mumbai. One curious thing is that some of the ancient symbols (for example, "7") look closer to Western modern digits than to the Indian symbols nowadays.
|
|
|
I have said that on the page pictured above, the numerals are dense. To illustrate this, I have overlaid the Bakhshali digits with modern ones in the following figure:

We shall see later where all these numbers come from. But right now I just want to point out that the sequences that seem to represent large numbers do in fact represent large numbers. The pair of numbers $1108 9512 5022$ on top of $1554 6150 00$ represents the fraction $$ {1108 9512 5022 \phantom{x} \over 1554 6150 00 \phantom{x} } \quad . $$
Even better, one can conjecture with near certainty that the sequence $5075 3383 7627 4674 327$ can be extended by $1936$, and the number $7250 4833 9467 5000 0$ can be extended by $00$. Together, these two make up the fraction $$ { 5075 3383 7627 4674 327 1936 \phantom{xx} \over 7250 4833 9467 5000 0 00 \phantom{xx}} \qquad ! $$ In fact, there are excellent grounds for conjecturing what all the missing numbers on the page are:

Here I have colored in green the digits one can see part of, and in red the conjectured ones.
The biggest number
The numbers on 46r are not the largest numbers in the manuscript, although they are nearly so. The largest number that appears is $26 53 29 62 26 44 70 64 99 42 83 21 18 7$, and it appears in a rather strange way. It is along the bottom of 58r:

Well, some of it is along the bottom. Where do the rest of the digits come from?

Here, as earlier, I have displayed in yellow the numerals that one sees clearly, in green those that one sees part of, and in red those that one conjectures. For the earlier page the numbers could be conjectured, with some work, because they are part of a mathematical computation that one can trace for oneself. That is not the case here. Instead, what is going on is perhaps a unique feature of Indian mathematics. Almost all of ancient Indian literature is in verse, and of course numerals do not exactly scan. Numbers are often written as words. Towards the top of 58r a sequence of words is found that records a single number as pairs of digits - for example, Sanskrit versions of "six-and-twenty" for $26$ and "one-less-than-thirty" for $29$---and the words at the top of the page read (but also with a few items guessed at) $26\;53\;29\;62\;26\;44\;70\;64\;99\;42\;83\;21\;18\;7$. There is no mathematics at all! Why this page is included in the manuscript is a mystery. As I have already mentioned, the Bakhshali manuscript is a collection of things with little connection to each other and no overall theme.
Square roots and arithmetical progressions
The basic pattern in the manuscript is this: (1) A rule (called a sutra) for solving a mathematical problem is stated or at least alluded to; (2) some examples of how the solution is carried out; (3) just after each example, some kind of check is run on the solution. The sutra is stated in words instead of an algebraic formula. There can be a great deal of arithmetic involved, but in general things are explained in such a way that one might as well be using algebraic variables.
One curious category of problems that occurs is this: one is given an arithmetic progression in terms of (i) its initial term $a$, (ii) the common difference between terms $\Delta$, and (iii) the sum $S$ of the series, and asked, how many terms are there in the progression? One learns somewhere in elementary education that $$ S = \left[ { (n-1)\Delta \over 2 } + a \right] n $$ and one can solve this quadratic equation to see that $$ n = { \sqrt{8\Delta S + (2a-\Delta)^{2}\phantom{x} } - (2a-\Delta) \over 2\Delta } \, . $$
For example, if I tell you that $\Delta = 3$, $a = 2$, and $S = 442$, then $2a-\Delta = 1$ and you can calculate $$ n = { \sqrt{8\cdot 3\cdot 442 + 1^2\phantom{x} } - 1 \over 2\cdot 3 } = 17 \, . $$
Furthermore, you can check to see that this correct by computing $$ \left( { (17-1)\cdot 3 \over 2 } + 2 \right) 17 = 442 \, . $$
But in the Bakhshali manuscript there generally arises something rather strange--the number of terms, which must surely be an integer if the problem is to make any real sense, is never found to be one. The motivation for these problems is therefore rather mysterious.
Finding the value of $n$ involves finding a square root. Now since the final $n$ is not an integer, the exact square root cannot be a rational number. You can only find an approximation to it. One of the most intriguing features of the manuscript is precisely its technique for finding approximations to square roots of integers that are not perfect squares. It almost seems that the person who made up these problems is far more interested in demonstrating his square-root approximation techniques than in posing problems with any real meaning. This is made more interesting because it was only somewhat later that Arabian mathematicians invented decimal fractions (like $1.414213562 \dots $), so that all work was to be done with ordinary fractions (like $665857/470832$). What is impressive to us is that the approximation technique involves some serious rational arithmetic. It is even more impressive that checking the correctness of the solution, involves some extremely serious rational arithmetic, as we shall see.
This sort of question about arithmetic progressions with non-integral $n$ occurs elsewhere in Indian mathematical treatises. Dealing with such very large numbers as the Bakhshali manuscript does is very rare.
In the Bakhshali manuscript, this sort of problem occurs on the pages 65v, 56vr, 64rv, 57vr, 45rv, and 46r (this illustrates that Hayashi's order is different from Kaye's). Unfortunately, these pages are damaged, and all lack certain parts of the computations. However, there remains enough that one can plausibly fill in the missing parts. This was attempted already by the first editor of the manuscript, G. R. Kaye, who understood the basic techniques involved but missed out on important details. These were added very soon after Kaye by the Indian historian of mathematics Bibhutibhusan Datta, and then finally the task was finished in the edition of Takao Hayashi. All in all, an impressive accomplishment.
The problem that produces page 46r begins on 45v, and has as basic data $$ a = 3/2, \quad \Delta = 3/2, \quad S = 7000 \, . $$
We then have $2a - \Delta = 3/2$ and get $$ \eqalign { n &= { \sqrt{ 8 \cdot (3/2) \cdot 7000 + (3/2)^{2} \phantom{x}} - (3/2) \phantom{x} \over 2 \cdot 3/2 } \cr &= { \sqrt{ 84000 + 9/4 \phantom{x} } - (3/2) \over 3 } \cr &= { \sqrt{ 336009 / 4 \phantom{x} } - (3/2) \over 3 } \cr &= { (\sqrt{ 336009\phantom{.}} / 2) - (3/2) \over 3 } \, . \cr } $$
Now $336009$ is not a perfect square. We would express it as $579.662833 \dots $ (the advantage of decimal fractions is that estimates of relative size are immediate). This option was not available to the author of the Bakhshali manuscript. Instead, with a little work he would see that $$ 336009 = 579^2 + 768 = 580^2 - 391 $$
and deduce only that $\sqrt{336009} = 579 + \hbox{ something between $0$ and $1$ } $.
What next? Nearly all civilizations had from early days a reasonable approximate formula for square roots, and the Indians were no exception. The common formula asserts that $$ \sqrt{ N^2 + A } \sim N + { A \over 2N } \, . $$
Since the days of Isaac Newton, we would derive it as the first terms in the series expansion $$ \sqrt{N^2 + A } = N \sqrt{ 1 + A/N^2\phantom{x} } = N \left( 1 + (1/2) \left({A \over N^2 }\right) + { (1/2)(1/2-1) \phantom{x} \over 2 } \left( { A \over N^2 } \right )^{2} + \cdots \ \right) $$
We don't have any idea how this approximation was first found in India, but what is intriguing is that the Bakhshali manuscript knows of an even better one. The elementary formula says that we have an approximation $p_{1}$ to $\sqrt{K}$ and $K = p_{1}^{2} + E_{1}$ with $E_{1}$ small so that $p_{1}$ is a first approximation to $\sqrt{K}$, then $$ p_{2} = p_{1} + { E_{1} \over 2p_{1} } $$
is a better approximation. In modern terms, the Bakhshali manuscript applies this yet one more time to get the approximation $$ p_{3} = p_{2} + {E_{2} \over 2 p_{2} } $$
with $E_{2} = K - p_{2}^{2} = -(E_{1}/p_{1})^{2}$. The manuscript doesn't say anything about derivation, it just tells you to calculate the following in order to approximate $\sqrt{K}$ for $K = p_{1}^{2} + E$: $$ \eqalign { p_{2} &= p_{1} + { E_{1} \over 2p_{1} } \cr p_{3} &= p_{2} - { (E_{1}/2p_{1})^{2} \over 2 p_{2} } \, . \cr } $$
In our case $K = 336009 = 569^2 + 768$, so $$ \eqalign { p_{1} &= 569 \cr E_{1} &= 768 \, . \cr } $$
Then $$ \eqalign { p_{2} &= 569 + { 768 \over 2 \cdot 569 } \cr &= { 2\cdot 569^2 + 768 \over 2 \cdot 569 }\cr &= {111875 \over 193} \cr E_{1}/2p_{1} &= { 768 \over 1138 } = { 128 \over 193 } \cr (E_{1}/2p_{1})^{2} &= { 294912 \over 777307500 }\cr p_{3} &= p_{2} - { (E_{1}/2p_{1})^{2} \over 2p_{2} } \cr &= { 12516007433 \phantom{x} \over 43183750\phantom{x} } \cr } $$ and we arrive at the impressive evaluation $$ n_{3} = { (p_{3}/2) - (3/2) \over 3 } = { 448244345088 \phantom{x} \over 4663845000 \phantom{x}} \, . $$
When tracking the computations in the manuscript, it is often necessary not to reduce fractions.
It is right at this point that 46r takes up the story. It is wholly concerned with checking the solution, i.e. checking that $$ S = \left( { (n-1)\Delta \over 2 } - a \right) n \, . $$
There are couple of things to note, however. (1) Checking isn't going to work, because we are not using an exact value for $\sqrt{K'}$ but only an approximation. (2) The number $n$ is quite large, and when we calculate $S$ we are going to find ourselves multiplying two large numbers to get an even larger one. To be precise, the numerator of $n$ has $12$ digits, so in the process of calculating $S$ we can expect to see around $24$ digits.
To deal with the first problem, we have to take into account the error between our approximation $p_{3}$ to $\sqrt{K}$ and the exact value. In fact, it comes to knowing the difference $K - p_{3}^{2}$, which happens to be a by-product of the work. As for the second, it is true that we wind up looking at a fraction with incredibly large numerator and denominator, but then a miracle occurs: $$ { 50753383 762725000000000 \phantom{xx} \over 7250483394675000000\phantom{xxx} } = 7000 \, ,$$
which is the value of the original $S$.
Tracking the author of the manuscript through all the necessary computations, first Datta and then Hayashi managed to fill in many of the missing parts of pages in the manuscript. In particular, 46r becomes

I'm not sure which I admire more, the original author or those who managed to reconstruct the manuscript. In any case, stunning performances.
My own personal belief is that the original work from which the Bakhshali manuscript originates was very close to the invention of the full decimal place system of arithmetic. I think what it really demonstrates is how exciting the invention of the decimal system--including "0"--was. Somewhat akin to the invention of computers: recursion is addictive. Being able to deal with every decimal place exactly the same as every other place is a very appealing notion. It certainly took some extraordinary level of enthusiasm to do all the arithmetic involved.
Which leads me to another remark. Historians of science often seem to write about their subject as if scientific progress were a necessary sociological development. But as far as I can see it is largely driven by enthusiasm, and characterized largely by randomness.
The present state of the manuscript
After the photographs of the manuscript were taken for Kaye's edition, all but one of them was encased in mica sheets and made into an album. The following image, taken from Kaye's book, shows what a typical pair of facing pages looked like around 1927.

The mica sheets were an unfortunate choice. The bark has stuck to them and broken up into hundreds and hundreds of tiny fragments. In addition, the bark has darkened considerably. It is now impossible to restore the manuscript in a good way, and at first sight you might think that taking new photographs of the manuscript would be out of the question. However, modern technology is wonderful, and the good news is that it is apparently possible to take good photographs of the pages even though they are extremely dark and encased in mica. You can see this in one of the recent photographs issued by the Bodleian Library.
How old is it?
Dating the manuscript has been an interesting problem ever since it was discovered. After a number of reasonable estimates and many more wild ones, Hayashi asserted that he thought that the copy of the manuscript that we now have was made around the eighth century, but that the original was probably much older. This was based on largely paleographic criteria. In the summer of 2017 Oxford University arranged to have some small pieces of bark, from 16, 17, and 33, dated in one of their physics laboratories. They came up with ranges of years 224-383 C.E., 680-779 C.E., and 885-993 C.E. There are many problems with these, principally that none of the experts sees any reason to think parts of the manuscript were made at widely different times. In particular, the material on 17r is clearly a direct continuation of that on 16v, and it is very hard to believe that it was written 400 years later. This, along with other objections, have been brought out in a recent paper by Kim Plofker, Agathe Keller, Takao Hayashi, Clemency Montelle, and Dominik Wujastyk. I am not aware that any resolution of difficulties is in sight.
It didn't help that Oxford University handled the event as more of an opportunity for a public media display than a scholarly research announcement. There was an accompanying video that seems to have been especially painful to those who were already acquainted with the manuscript.
Acknowledgements
The photographs of pages of the Bakhshali manuscript were made from the images in G. R. Kaye's edition.
I wish to thank S. G. Dani for making some of the more obscure literature available to me, and for several helpful suggestions. Also Takao Hayashi for having the patience to answer my naive questions.
- August Hoernle, On the Bakhshali manuscript, Alfred Hölder, Vienna, 1887.
Hoernle, who at this time worked for the Government of India, was the first expert to see the manuscript.
- G. R. Kaye, The Bakhshali Manuscript: a study in medieval mathematics, the Archaeological Survey of India, Calcutta, 1927.
Many of Kaye's opinions are clearly wrong, but parts of this are readable and interesting. His book contains the only good publicly available images of the pages of the manuscript. I have made photographic copies of all of these, and posted them in various sizes at
http://www.math.ubc.ca/~cass/bakhshali/.
- Bibhutibhusan Datta, "The Bakhshali mathematics," The Bulletin of the Calcutta Mathematical Society 21, 1-60.
This criticizes Kaye's edition severely. It is also a good introduction to the Bakhshali manuscript, even if occasionally itself in error as well as incomplete.
- Takao Hayashi, The Bakhshali Manuscript: an ancient Indian mathematical treatise, Groningen, 1995.
This is the definitive account.
- A press release from the Bodleian Library.
This includes photographs of one of the folia (to which I imagine they have applied some image manipulation to enhance contrast), as well as a photograph of the album of mica-encased bark fragments. You can see two of the facing pages in this album in Kaye's Plate I reproduced above.
- Article in The Guardian about the Bodleian's announcement.
This was just one of several exaggerated press announcements.
- Kim Plofker, Agathe Keller, Takao Hayashi, Clemency Montelle, and Dominik Wujastyk, The Bakhshali Manuscript: A response to the Bodleian Library's radiocarbon dating, History of Science in South Asia 5 (2017).
Critical of the Bodleian announcement.
- Map of modern Bakhshali and neighbourhood.
- A treatise on the manuscript, together with a transcription into a more widely known dialect, compiled by U. Jyotishmati and S. S. P. Sarasvati. Published in Allahabad, 1979. The historical introduction has things no other source does.
- The Wikipedia entry on birch bark manuscripts.
- The Wikipedia entry on the manuscript.
- J. L. Berggren, Episodes in the history of medieval Islam, Springer, 2003.
Section 2.3 has a brief and valuable account of the invention of decimal fractions.
 Bill Casselman
Bill Casselman
University of British Columbia, Vancouver, Canada
Email Bill Casselman


 Bill Casselman
Bill Casselman






