Math and the Musical Offering

g(t) = f(18-t) and Canon 1

Perhaps the most exotic canon in the collection is the first: Canon 1, Cancrizans. It is also the simplest.

Graphic © 1996, Timothy A. Smith, used by permission.

``Cancrizans'' means ``crabwise'', but in fact in this canon Voice 2 plays the score of Voice 1 backwards. This is indicated in Bach's cryptic presentation by the backwards signature at the end of the piece. Voice 1 plays the ``royal theme'' itself (8.5 measures), followed by 9.5 measures of counterpoint.

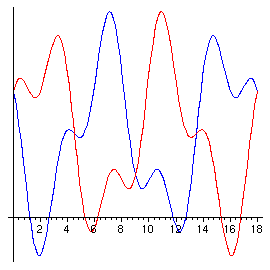This score is eighteen measures long. For a function g defined on that interval to be ``backwards'' of f, we need g(0) = f(18), g(1) = f(17), etc. This is achieved by defining

In this plot,as usual, f  is the red graph and g is the blue, althoughin this case the operation is symmetric, and f runs g backwards also.
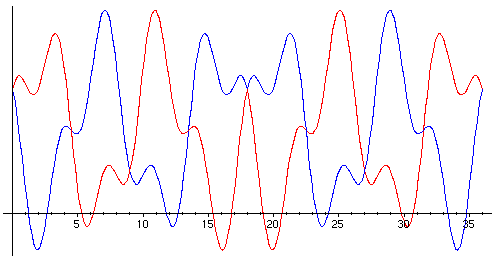
Usually in performance each player plays his or her score forwards and then backwards. (If the instruments are different, this is not the same as running through the piece twice.) This results in the pattern: