
Mathematical ImageryMathematical artists create strong, stunning works in all media and explore the visualization of mathematics
2016 Mathematical Art Exhibition
The 2016 Mathematical Art Exhibition was held at the Joint Mathematical Meetings held in Seattle, WA. Here on Mathematical Imagery is a selection of the works in various media, including recipients of the 2016 Mathematical Art Exhibition Awards: "45 Poppies," by Karl Kattchee was awarded Best photograph, painting, or print; "Sword Dancing," by George Hart was awarded Best textile, sculpture, or other medium; and "OSU Triptych No. 2," by Robert Orndorff received Honorable Mention. The Award "for aesthetically pleasing works that combine mathematics and art" was established in 2008 through an endowment provided to the American Mathematical Society by an anonymous donor who wishes to acknowledge those whose works demonstrate the beauty and elegance of mathematics expressed in a visual art form.

Printed polyester crepe de chine, bead crochet (glass beads and thread), 70 x 50 cm, 2015. This infinity scarf and bead crochet necklace are twin tori. The fabric design is (an elongated version of) the infinitely repeating planar pattern that a tiny explorer could map by charting the surface of the necklace in all directions (the universal cover of the beaded rope). The two colors, identical tessellated wave motifs, gradually transform from "calm" to "busy." The pattern at each step has an increasing "busyness" quotient (a measure of how much the individual beads in a fundamental tile differ in color from neighboring beads). The scarf, sewn from a parallelogram to create a mobius-like twisted torus, has a small hole in one seam so that it can be turned inside out to explore the puzzling behavior of torus inversions. --- Ellie Baker

Knitted wool (various sources) and printed photographs, 30 x 30 x 7 cm, 2015. The central property of the Borromean rings--that removing any component unlinks the remaining components, which collectively form the unlink--generalizes to the class of Brunnian links. The Rainbow Brunnian Link Cowl has seven components rather than the three components of the Borromean rings. All linking is intrinsic, rather than introduced post-construction via grafting. The Rainbow Brunnian Link Cowl is also a garment that can be worn two different ways, which are pictured alongside that cowl in the exhibit. --- sarah-marie belcastro

Lasercut woods, 15 x 45 cm, 2015. The Jordan Curve Theorem states that when a simple closed curve is drawn in the plane, it will cut the plane into two regions: the part lies inside the curve (here, the slightly darker-colored inset piece of wood), and the part that lies outside it (here, the slightly brighter and thicker frame). --- Robert Bosch

Merino Wool Yarn, 137 x 18 cm, 2015. This double knit scarf brings together the recursive construction of a fractal, the dragon fractal, as well as the recursive construction of an integer sequence, the Fibonacci sequence. The main panels of the scarf are based on a pattern developed from the eleventh iteration of the dragon fractal. Moreover, the striping pattern in between the main panels is illustrative of the Fibonacci sequence with color changes after 1 row, after another 1 row, after 2 rows, after 3 rows, after 5 rows, and with another color change after 8 rows. As this is a double knit pattern, the back side of the scarf is shown in the reverse color pattern. --- Rachelle Bouchat

KnitPicks Shine Sport yarn: 60% cotton 40% modal, 7 x 44 x 44 cm, 2015. "Hyperbolic Afghan {3, 7}" illustrates a tiling of the hyperbolic plane by triangles, 3 at a vertex, in crocheted cotton. Adapting techniques developed by Joshua and Lana Holden, the piece is not assembled from flat triangles but instead approximates constant curvature over its entire surface. Its coloration, inspired by William Thurston's rendition of the heptagon tiling underlying the Klein quartic, suggests the identifications required to construct that surface as a quotient of the hyperbolic plane. --- Heidi Burgiel
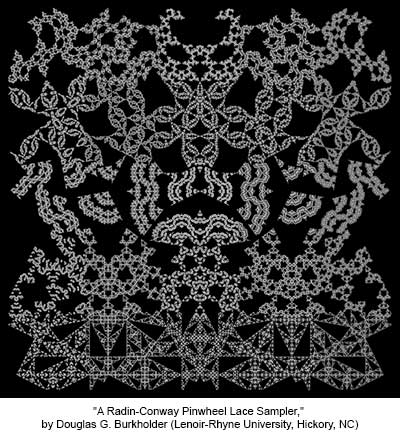
Digital Print, 50 x 50 cm, 2015. This artwork evolved from a search for beauty and patterns within Conway and Radin's non-periodic Pinwheel Tiling of the plane by 1x2 right triangles. The Pinwheel tiling can be created by repeatedly subdividing every triangle into five smaller triangles. This lace resulted from alternately subdividing triangles and removing triangles. Triangles are removed based upon their location in the next larger triangle. First, on the macro level, the five distinctive removal rules are applied one to each row. This removal rule is especially easy to see in the bottom row. These same five rules are then applied, on the micro level, to the columns. The remaining triangles form a sampling of twenty-five styles of lace generated by the Pinwheel tiling. --- Douglas G. Burkholder
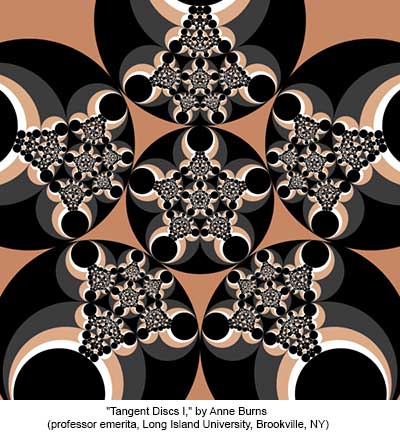
Digital print, 30 x 30 cm, 2015. I am interested in the connections between mathematics, art and nature, especially the concept of evolution. Thus my mathematics interests are dynamical systems, differential equations and any area that deals with states that evolve with time. This image is an iterated Function System consisting of a group of (six) Möbius Transformations acts on six discs, five of which are tangent to the unit circle, to its two neighboring discs and to a sixth disc centered at the origin. --- Anne Burns

Archival inkjet print, 60 x 60 cm, 2015. This image presents a visualization of the Steiner triple system S(2,3,7). This system, which is combinatorially equivalent to the Fano plane, consists of seven three-element subsets (or blocks) drawn from a seven element set such that any pair of elements occur in exactly one block, and any two blocks have exactly one element in common. --- Conan Chadbourne

Mathematics and mathematical visualizations are meaningful at many scientific and technological levels. They are also an endless source of inspiration for artists. The following artworks are part of the 12-30 project ? one mathematical image a day for one year, 12 mathematical visualization software, January 1st, 2015 ? December 31, 2015. See the 365 images portfolio. --- Jean Constant

Manipulation of digital images, and use of ray-tracing software can help you to concretize mathematical concepts. Either for giving you an idea of how a real object will look or to represent imaginary landscapes only computers can handle. Here, ring cyclides are images of tori under sphere inversion. If certain conditions are fulfilled, a torus can contain a set of tangents spheres. Since the tangency property is preserved by inversion, this set of tangent spheres find its place inside the cyclide. --- Francesco De Comité

Printed cardboard, 50 x 30 x 30 cm, 2015. The goal of my art is to create aesthetically pleasing repeating hyperbolic patterns. One way to do this is to place patterns on (connected) triply periodic polyhedra in Euclidean 3-space. This polyhedron is constructed by placing regular octahedra on all the faces of another such octahedron, so there are 12 equilateral triangles about each vertex. Each of the triangular faces has been 90% filled by a fractal pattern of circles provided by John Shier. The polyhedron consists of red and blue "diamond lattice" polyhedra and purple octahedra that connect the red and blue polyhedra. Each of the red and blue polyhedra consists of octahedral "hubs" connected by octahedral "struts", each hub having 4 struts projecting from alternate faces. The red and blue polyhedra are in dual position with respect to each other - they form interlocking cages. Each purple connector has a red and a blue octahedron on opposite faces. --- Doug Dunham

Aluminum print, 51 x 61 cm, 2015. My artistic impulse is to let the beauty of the real world shine into the realm of mathematical patterns. My method combines photographs with complex-valued functions in the plane to create images with all possible types of symmetry. I photographed the irises and used complex wave functions to turn the image into a pattern with four-fold rotational symmetry. Then I applied a complex exponential mapping to wind the wallpaper around the complex plane, choosing just the right scaling to make the pattern match, while also creating five-fold symmetry. I bleached an outer ring to bring focus to the center of the spiral and to allow the original photograph of the iris to stand out. Details about wallpaper waves appear in my book, Creating Symmetry: The Artful Mathematics of Wallpaper Patterns. --- Frank A. Farris

Ceramics, 60 x 45 x 3 cm, 2014. I'm endlessly fascinated by certain aspects of our world, including symmetry, chaos, and infinity. Mathematics allows me to explore these topics in distinctive artworks that I feel are an intriguing blend of complexity and beauty. This sculpture is based on a particular stage in the development of a fractal curve known as the ternary dragon. This ceramic piece has been mounted on a board, with standoffs, partly to make it easier to handle without breaking. The resulting construct could be viewed as either a two-dimensional or three-dimensional artwork, which echoes the manner in which fractal curves can be considered as one-dimensional (a line), two-dimensional (a plane-filling object), or something in between. --- Robert Fathauer

Strips of polypropylene ribbon, 13 x 10 x 10 cm, 2013. Loosely defined, a 'Buckyball' is a polyhedron made of pentagons and hexagons with every vertex of degree three (three edges meeting). Buckyballs must have exactly twelve pentagons. I enjoy creating Buckyballs and their duals. I discovered that if you rearranged the twelve pentagons in a semi-regular pattern you could get interesting shapes. Thus began my series of eggs. --- Faye Goldman

Merino yarn, cotton thread, embroidery hoop, 21 x 26 x 26 cm, 2015. For me, the most exciting part of mathematics is communicating it to others. I am especially interested in models that make mathematical concepts tactile or visual. In Fibonacci Downpour, the vertical stitch lines branch and form drops following a physical version of the Fibonacci recursion. The number of drops and branchings in each row are consecutive Fibonacci numbers. As the Fibonacci numbers are asymptotically exponential, the fabric falls into a more or less pseudospherical form. --- Susan Goldstine

Laser cut paper, 20 x 20 x 20 cm, 2014. I like to play with the ways that the arts can reveal the often hidden beauty of mathematics and that mathematics can be used to produce interesting or beautiful art. 32 pieces of paper cut into two shapes connect and weave together to form a ball mixing the dodecahedron and icosahedron. Inspired by Quintron by Bathsheba Grossman. --- Edmund Harriss

Cotton cross-stitch, 38 x 38 x 5 cm, 2009. I am fascinated by mathematical patterns and enjoy using them to make something useful. Cushion with each tile shape for the Ammann-Beenker tiling in a different colour. --- Maggi Harriss

Best textile, sculpture, or other medium, 2016 Mathematical Art Exhibition
Wood (dyed) and cable ties, 32 x 45 x 45 cm, 2015. As a sculptor of constructive geometric forms, my work deals with patterns and relationships derived from classical ideals of balance and symmetry. Mathematical yet organic, these abstract forms invite the viewer to partake of the geometric aesthetic. This is a model for a large wood sculpture consisting of two congruent but mirror-image orbs of this design, each two meters in diameter. The sixty components of the design are "affine equivalent," meaning they can be stretched linearly to become congruent to each other. They lie in groups of three in twenty planes--the planes of a regular icosahedron which had been compressed by a factor of 1/2 along a five-fold axis. --- George Hart

Stainless steel wire, shell, driftwood cedar frame, 40 x 36 x 9 cm, 2015. "Waves" was designed and created by lenka using a tessellation pattern generated algorythmically by Veronica. Bobbin lace, a 500-year-old art form, features delicate patterns formed by alternating braids. Lenka: "I had a beautiful frame made from old growth, driftwood red cedar and I needed a pattern that would look like the waves of the Pacific Ocean... The model is an incredible source of designs--every graph has so many variations for working the stitches and each combination results in a different pattern. I love the experimental nature of the work. --- Veronika Irvine & Lenka Suchanek
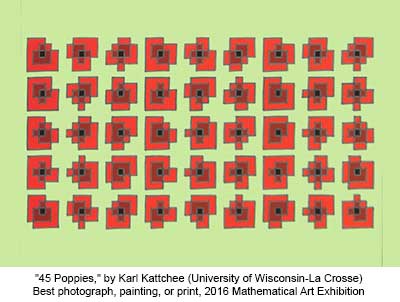
Best photograph, painting, or print, 2016 Mathematical Art Exhibition
Digital print, 18 x 31 cm, 2015. This image is a classification of all closed paths, on a 6x6 grid, with the following properties: First, each path must proceed around the center of the grid and be orthogonal in the sense that every turn is 90 degrees. Also, the path must use each row and column exactly once. Finally, we require that each path be asymmetrical, and we do not distinguish between paths which differ by a rotation or flip. Each center square is colored black, and the shades of red are dictated by the winding number of each region. Acknowledgements: Craig Kaplan (Waterloo), for helpful notation and the coloring scheme idea, and artists Kate Hawkes and Misha Bolstad (UW-La Crosse) for the poppies idea. --- Karl Kattchee

Archival inkjet print, 40 x 60 cm, 2015. I enjoy exploring the possibilities for conveying ideas in new ways, primarily visually. I have a background in mathematics, which provides me with a never-ending supply of subject matter. The Catalan numbers are a sequence of positive integers that provide answers to certain combinatorial questions. For example, in how many ways can a polygon with n+2 sides be cut into triangles? A hexagon (setting n=4) can be triangulated in fourteen different ways, so the 4th Catalan number is 14. Other types of problems also lead to the Catalan numbers: counting binary trees, balancing parentheses, finding paths through a grid, shaking hands in a circle, etc. This piece is composed of diagrams representing seven different problems; for each of these, the answer is the 4th Catalan number. The solution sets for the problems are displayed in diagonal bands. The columns indicate correspondences between elements in different solution sets. --- Margaret Kepner

Watercolor paper, acrylic paint, polyester thread, 38 x 38 x 38 cm, 2014. I create forms art of diagonal twill and structures not normally found in the basketry world. My medium for this unique work is watercolor paper, which I've painted and cut into very narrow uniform strips to achieve the precision I seek. I am very much interested in the math and geometric constraints of the work. Using hundreds of strips of paper at a time, I explore new structural forms. An interpretation of a hexahedron using diagonal twill to form a woven sculptural basket. --- Dorothy McGuinness

Photograph, 2016. I like to crochet hyperbolic surfaces. This object started as a hollow ovoid, top and bottom missing. I then crocheted three vertical axes down the sides. These three axes are the basis for the hyperbolic crochet. The object is hung from the ceiling. I then entered a light tube. The photograph was taken in the dark without flash. --- Gabriele Meyer

Digital print on aluminum panel, 40 x 40 cm, 2014. My work is composed primarily of computer generated, mathematically-inspired, abstract images. I draw from the areas of geometry, fractals and numerical analysis, and combine them with image processing technology. This image was created using a dynamic version of the Chaos Game algorithm. The Chaos Game is a simple example illustrating chaotic motion and strange attractors. It is typically implemented using three anchor points, which become the vertices of a Sierpinski triangle. The Dynamic Chaos Game allows the anchor points to move each iteration. Here, the image comprises seven panels. In each, the three anchor points slid along line segments, with different speeds. The pixels were then colored according how frequently that point was visited during the iteration. --- Kerry Mitchell

Honorable Mention, 2016 Mathematical Art Exhibition
Paper and acrylic, 20 x 46 cm, 2015. My paper folding art is math plus paper. Folded paper is simply tangible math. This is a permanent manifestation of an ephemeral artwork, namely, one solution for a specific one-straight-cut problem. Such problems are usually stated as follows: How must one fold a paper rectangle into a flat figure such that one straight cut through all of the layers will produce a given planar straight-line graph? Here the problem has been solved with paper and then represented in acrylic. To a significant degree the work relies on transmitted and reflected light, and so it never looks the same twice. The figure (the letters "OSU") has been divided into three frames. The crease patterns for the left and right letters are pedestrian but that for the central letter is sublime. --- Robert Orndorff

Walnut veneer and brass fasteners, 38 x 38 x 38 cm, 2015. I am interested in creating patterns that convey messages at multiple levels and scales using a wide variety of mathematical elements and media. This form is based on the small rhombicosidodecahedron, an Archimedean solid with 120 edges. The underlying polyhedral edges have been replaced by 4.75 cm squares made from laser-cut paper-backed walnut veneer and connected at their corners with brass split-pin fasteners. The 62 faces (squares, hexagons, and pentagons) and 60 vertices of the underlying polyhedron are transformed into open negative space. The expansion of linear edges into squares results in a sphere-like shape with 20 knobs. --- David Reimann

Back stitch embroidery on 18 point canvas, 31 x 31 cm, 2013. I have been working with needle crafts since graduate school. I have also been interested in fractals and fractal geometry for more than 20 years. The Levy Dragon Outside Tapestry consists of four copies of the Levy Dragon built from the edges of a square. The iteration steps are repeated on each of the four sides of the square with the initial triangle motif placed outside the square. This back stitch design shows the twelfth iteration for this outside construction.The outside tapestry was done in two shades of blue to better show each of the four copies of the Levy dragon. --- Larry Riddle

Tile, 50 x 50 cm, 2015. I am interested in Persian geometric art and its historical methods of construction. Kokabi Star (the great pentagram) can be constructed using the lines of the 10/3 star polygon. Patterning this star can be achieved using different approaches. Some of the presented stars in this artwork have been made based on the actual tiling on existing buildings. Some others have been constructed based on old treatises and scrolls. Some of the patterns have been created using the traditional compass-straightedge process. Modularity is another approach in this regard. Moreover, the two decorated quasicrystal patterns of Star and Sun (the only two quasicrystal patterns with global five-fold rotational symmetry) and their striking relationships with Kokabi Star have been presented. Is this relationship a theorem? --- Reza Sarhangi (1952-2016)

3D printed nylon plastic, lamp, 10 x 9 x 9 cm, 2014. The light rays from the lamp are partly blocked by the shrinking design on the sphere; the resulting shadow is a regular tiling of the plane by squares. This illustrates how stereographic projection transforms the sphere, minus the north pole, into the plane. Note how shapes are slightly distorted near the south pole, and dramatically distorted near the north pole. --- Henry Segerman

ABS plastic, printed on an FDM machine, 16 x 20 x 14 cm, 2015. Stimulated by the LEGO-Knot project, I aimed to design a set of modular parts that permits to compose not only various handle-bodies, but also single-sided surfaces of higher genus. The modular parts employed in my sculptures are tubular 3-way junctions, where one of the tubular stubs exposes the opposite side of the surface shown by the other two stubs. Depending on how the parts are connected, the resulting compositions remains orientable or becomes single-sided; in the latter case they correspond to sums of multiple Klein bottles; which I call "Super-Bottles." The two identical parts of which the sculpture is composed can be put together in three different ways. In two cases the resulting surface is single-sided ($\sigma = 1$) and in the third case it is double-sided ($\sigma = 2$). The genus of the resulting surface is $4/\sigma$ . The configuration shown is a non-orientable surface of genus 4, corresponding to the connected sum of two Klein Bottles, with two punctures. The insets show the two individual parts, and an assembly of them resulting in a 2-hole torus of genus 2 (with two punctures). --- Carlo Séquin


