
Mathematical ImageryMathematical artists create strong, stunning works in all media and explore the visualization of mathematics
Seifert Surfaces :: Jarke J. van Wijk
Some years ago, my colleague Arjeh Cohen asked me if I could visualize a Seifert surface. I quickly became fascinated with these strange and difficult surfaces. Seifert surfaces are orientable surfaces, bounded by a knot or link. Their shapes are real-world and not abstract, but also strange and difficult to comprehend. Therefore, I developed a tool called SeifertView to define and display these fascinating mathematical objects.
— Jarke J. van Wijk

The yellow tube is a (1, 3, 5) pretzel knot. Such a pretzel knot or link consists of a sequence of angles, where each tangle has a number of twists. The brown surface is a Seifert surface: an orientable surface bounded by the knot. Here the surface is easy to understand; for arbitrary knots such surfaces often have strange and difficult shapes. However, for any knot or link such surfaces can be found, as shown by Herbert Seifert in the 1930's. This image was made with a tool called SeifertView.
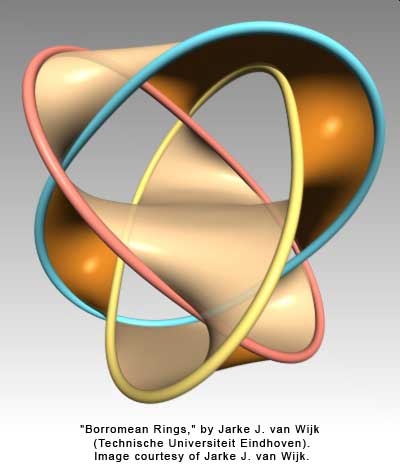
The Borromean Rings consist of three links. Take one link away and the other links fall apart, but together they are inseparable. Because of this, they are popular as a symbol for strength in unity. Here they are shown from an unusual point of view, and also a Seifert surface is shown. This is an orientable surface, bounded by the links. This image was made with a tool called SeifertView.

This knot consists of three similar links, and is threefold-symmetric. The surface shown is a Seifert surface, an orientable surface bounded by the links. Considering only the links, it is hard to imagine that such a surface does exist. However, in the 1930's, the German mathematician Herbert Seifert presented an algorithm to find such surfaces for any knot or link. This image was made with a tool called SeifertView.



