
AMS MathViewer
An interactive, dual-panel reading experience for AMS journals
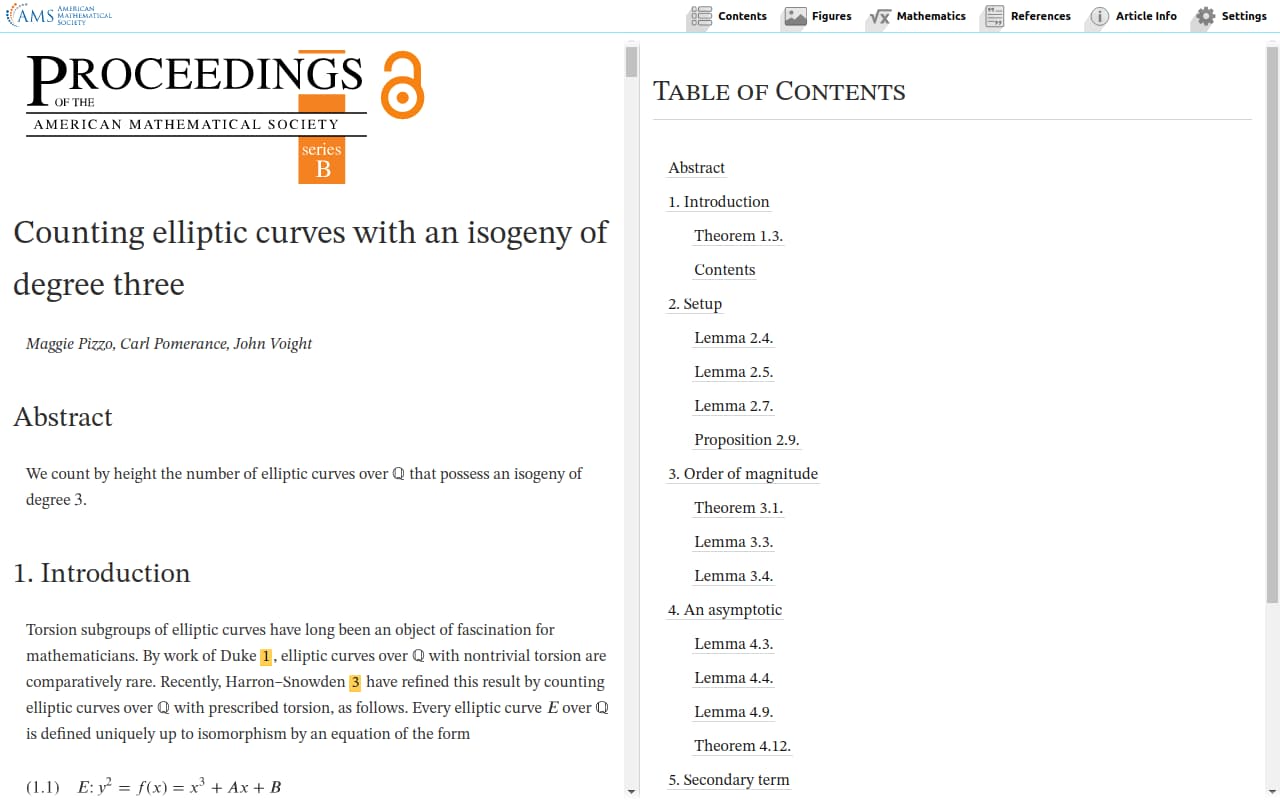
AMS MathViewer provides an option for viewing articles directly in your browser as full text HTML, offering an interactive alternative to PDF and print reading.
MathViewer's responsive design perfectly renders mathematical content on all devices. Click on embedded links to formulas, theorems, figures and references to see them in the second panel, right beside the original text. Two-way linking means you won't lose your place in the text.
AMS MathViewer articles work offline automatically – after your first visit, you can access the article again under any network conditions. Create a bookmark or use the "Add to Homepage" feature of your browser for easy access to an article.
Check out our deep-dive into AMS MathViewer features below!
Select the "View in AMS MathViewer" link, found on journal volume and article abstract pages, to use AMS MathViewer for the following journals:
- Proceedings of the American Mathematical Society - Series B
- Transactions of the American Mathematical Society - Series B
- Journal of the American Mathematical Society (Starting with the 2018 volume and for selected past articles)
- Mathematics of Computation (Starting with the 2019 volume and for selected past articles)
- Bulletin of the American Mathematical Society (featured articles, starting with the 2020 volume)
- Communications of the American Mathematical Society (launching 2021)
AMS MathViewer features
AMS MathViewer provides a simple, dual panel layout.
The primary panel contains the main article content while the secondary panel provides different content from the article such as references, figures, and article information. These two panels are visually presented side-by-side or vertically stacked, depending on your browser window.
The secondary panel also serves to present cross-referenced parts of the article without disruptinng the position in the primary panel.

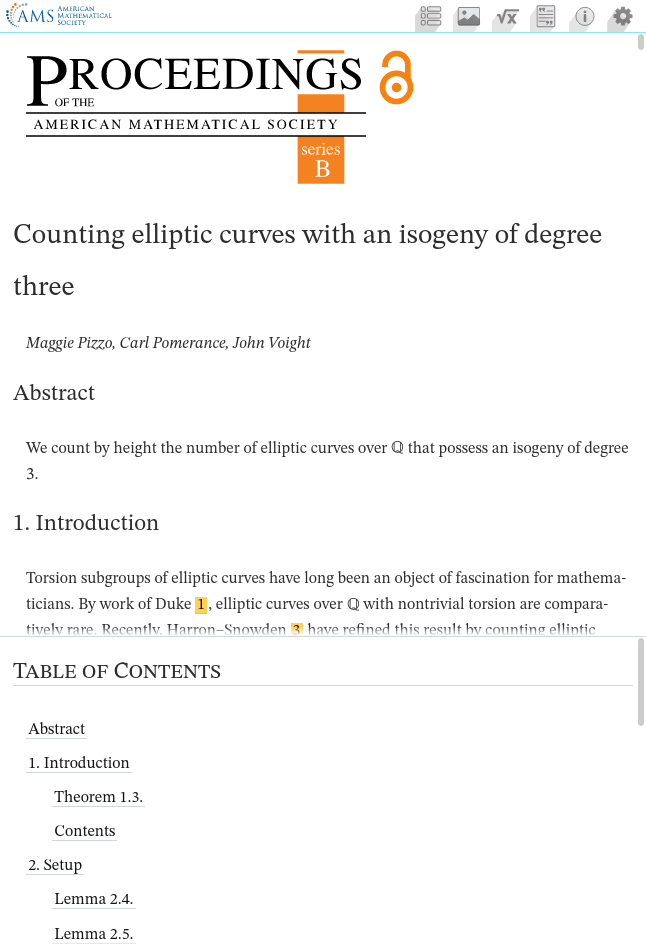
The main panel
AMS MathViewer is focused on providing a remarkable reading experience. The main panel displays the core article content in a clear typography using the STIX Two fonts. The layout combines print and web design traditions to the benefit of the reader.
Section headings, figures, and theorem environments are easy to spot even when scrolling rapidly while the core typography enables dedicated reading.
Never lose your reading position: Following cross-references to the bibliographic items, figures, or mathematical fragments such as numbered equations and theorems does not disrupt the reading flow; instead referenced content is displayed side-by-side in the secondary panel. Backlinks in the secondary panels allow the reverse, too!
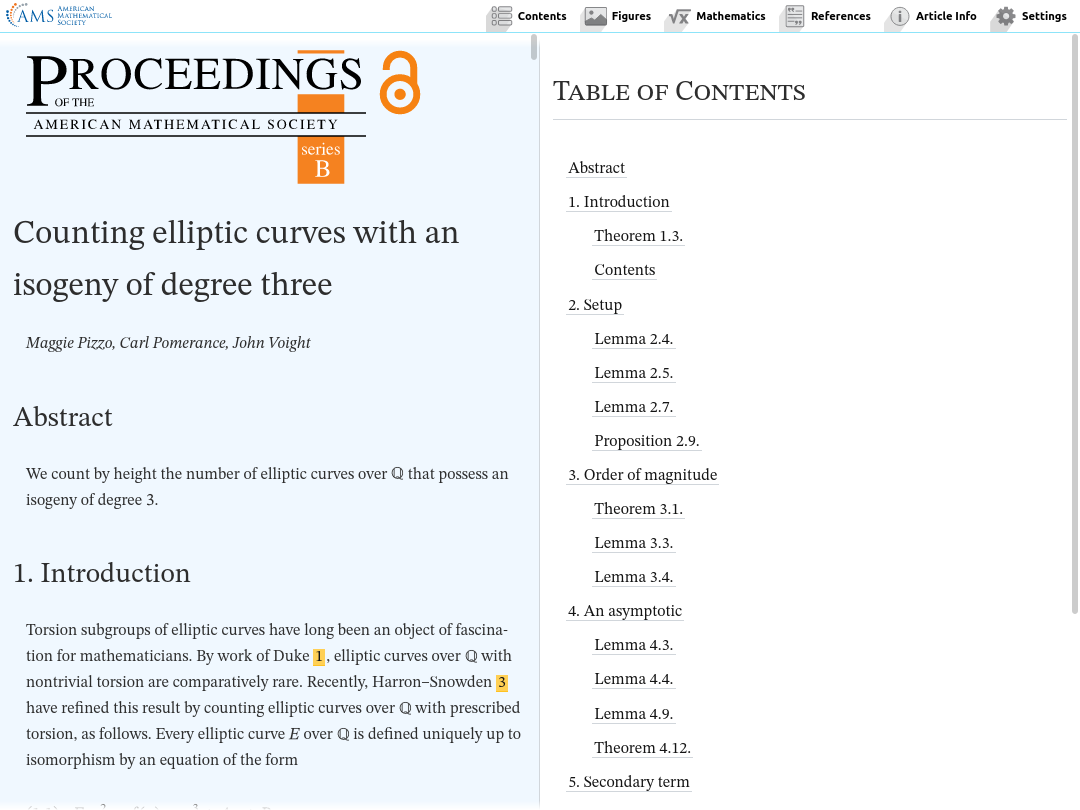
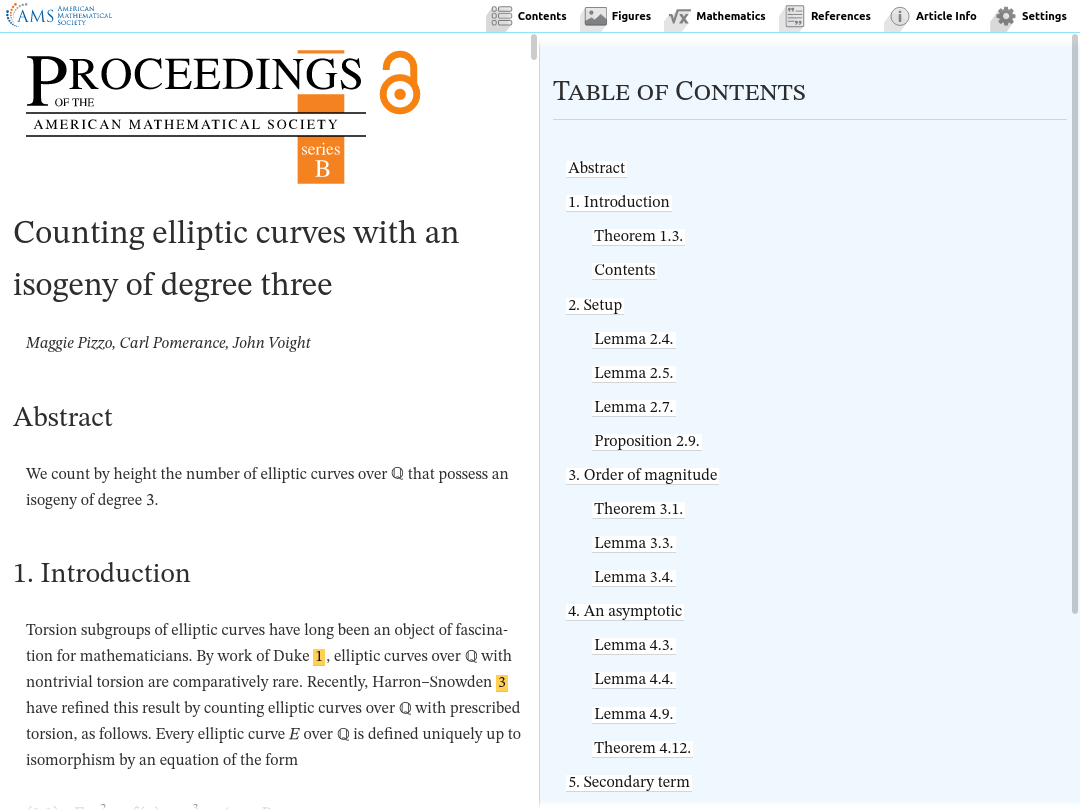
The TOC panel
The Table of Contents panel helps just as you would expect. Additionally, AMS MathViewer also includes theorems and lemmata to help you access them more quickly.
The Math (fragments) panel
The Math panel provides a copy of all cross-referenced equations and theorem environments. This allows readers to view a reference to such fragments side-by-side without losing the position of the main article content. As a separate panel, it does not restrict readers to just one reference but simplifies looking through other referenced content.

The Figure panel
The figure panel includes all referenced figures and tables. If the figure was not critical to the flow of the main content, it may only appear here. Otherwise, it is duplicated.

The References panel
The references panel presents the bibliography (and sometimes bibliographies) in a concise manner. Besides easy side-by-side view, the references provide access to DOIs, MR Reviews and AMSRefs data for each item. Backlinks of each item enable two-way exploration of the article.

The Article Information panel
The article information panel provides available article metadata. Besides the article metadata such as DOI, MR number and AMSRefs data, author and contributor information is provided. Additionally, the article's and journal's publication history as well as MSCs, keywords and other notes can be found here.
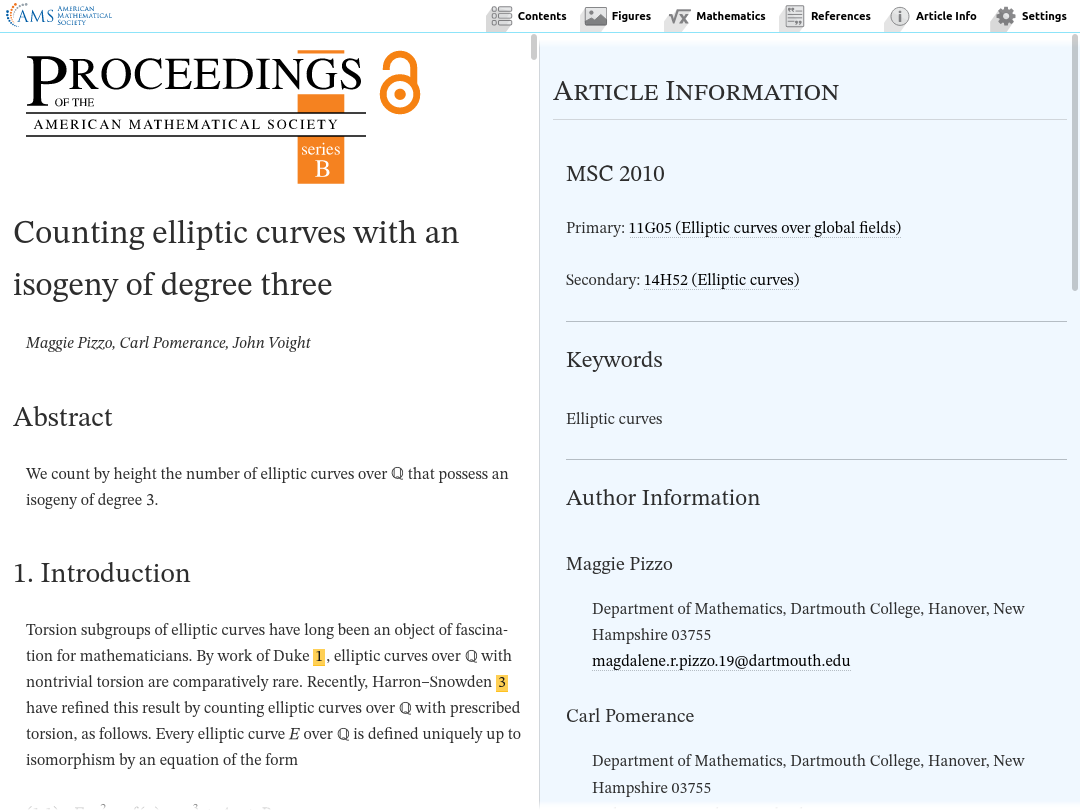
The Settings panel
The settings panel provides several features to customize the reading experience.
Readers can change the font-size (in addition their browser default settings and zoom).
Readers can customize the panel sizes (decreasing / increasing the article and secondary panel size).
Readers can activate subtitling and speech synthesis for equation exploration (see also the section about MathViewer's Accessibility Features). The availablility and quality of speech synthesis will depend on the browser and operating system as high quality synthetic voices may not be available.
Note that screenreader users should not activate speech synthesis to avoid duplicate voicing.

Accessibility
We consider accessibility as the heart of a good reading experience for all users. Formally speaking, AMS MathViewer aims to provide WCAG 2.1 AA-level compliance but we do not stop there - we continuously revise and extend MathViewer's design and functionality to enhance the experience of all users, but in particular for users of assistive technologies. Given the nature of mathematical content, this can be challenging. Our continuing efforts allow us to support more and more types of content in an accessible manner.
A particular goal for MathViewer is to provide robust, explorable equation layout. By leveraging MathJax and Speech Rule Engine in a unique way, we are able to create a powerful solution.
AMS MathViewer progressively enhances a reliable textual alternative that will always work, adding a full exploration when possible. This exploration mode leverages the established ARIA Treeview pattern which works in most combinations of browsers and assistive technologies, e.g., NVDA or JAWS on Windows, VoiceOver on Mac OS, Orca on Linux.
The exploration is available whenever an equation element is in focus (e.g., tabbed to, clicked on or after pressing ENTER when passing by in a screenreader's browse/virtual mode). Once in focus, users can use the arrow keys of a keyboard. (Support for touch input is on our roadmap.)
The text alternative primarily uses Speech Rule Engine's MathSpeak implementation. In addition, we provide simultaneous Nemeth Braille using an upcoming ARIA feature for braille-specific text alternatives; support in assistive technologies for this not-yet-official standard is currently limited.
This benefits users of assistive technology as well as all other users (see the section about MathViewer's Settings Panel).

Progressive enhancements
MathViewer is designed with both progressive enhancements and graceful degradation in mind. This means we will use web technologies in a way that enables better functionality on more capable systems without losing functionality on less capable systems. For example, MathViewer will provide a robust (albeit less powerful) presentation even without JavaScript and the visual design will not fail but be reduced on older browsers.
No more loss of access while traveling. MathViewer articles will work offline, that is, once visited in a modern browser the page is available independently of network conditions.
MathViewer articles can be "installed" on mobile homescreens which is enables additional benefits, e.g., easier access and full-screen display on mobile decvices.
Equation and reference sources
MathViewer provides both equation and references as raw data embedded in the article markup. When selecting text that includes equations, regular copy&paste will copy the text together with LaTeX sources for the equations.
For bibliographic items (in the References Panel) and for the article itself (in the Article Info Panel) you can access raw AMSRefs data via the AMSRef link.
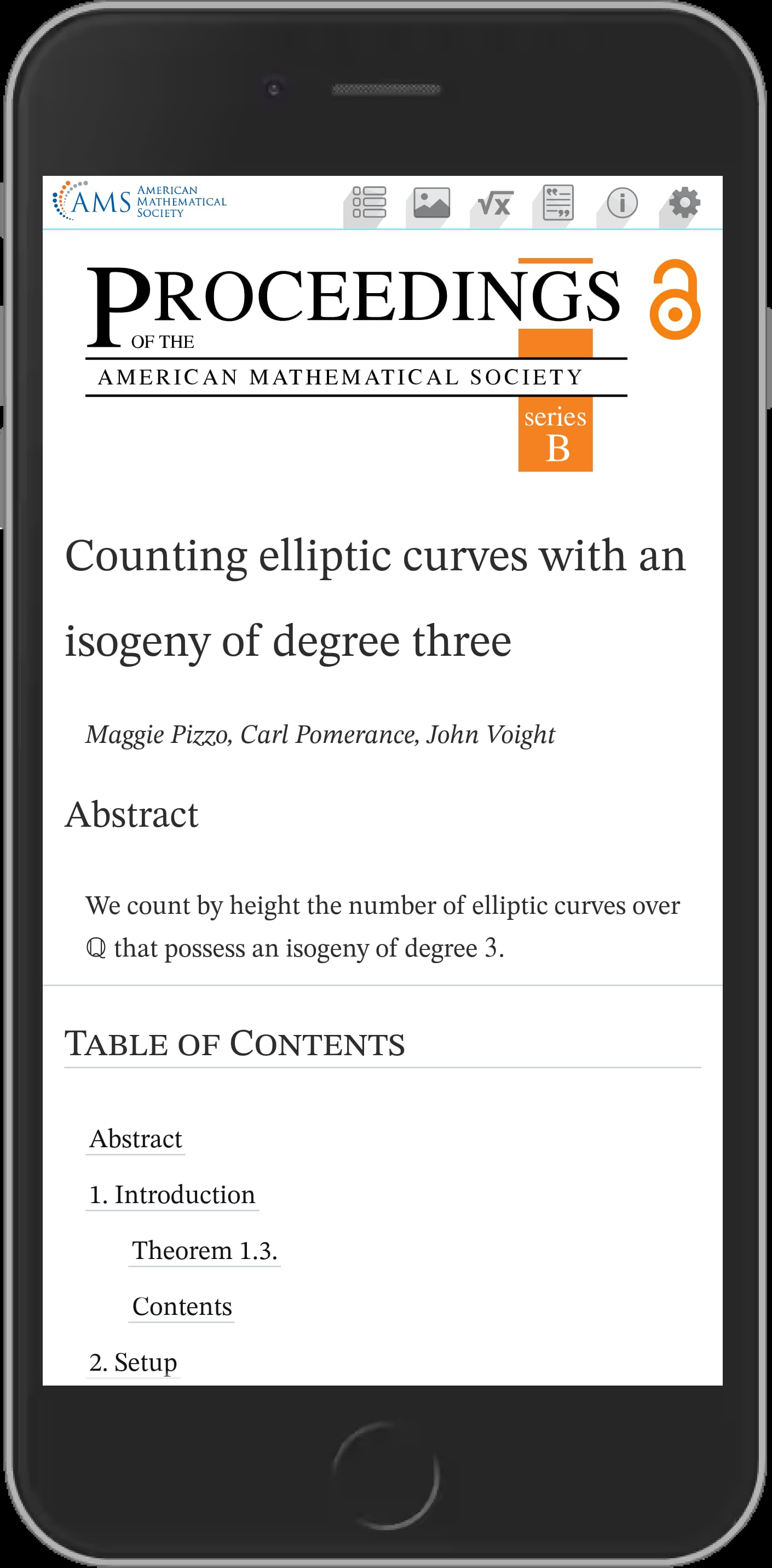
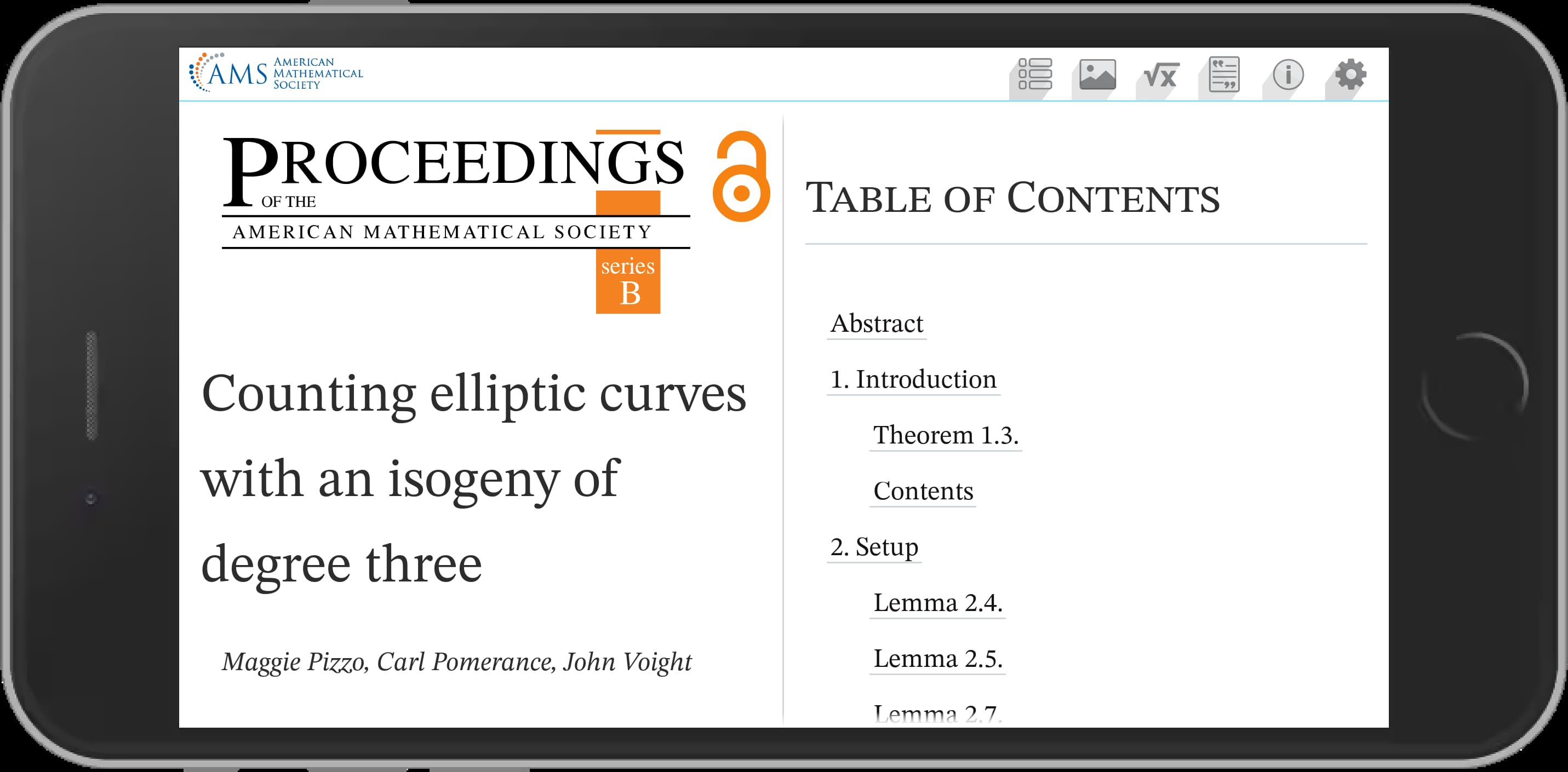
Explore articles on your phone or tablet
AMS MathViewer is designed to beautifully render across screen sizes. On narrow screens, the dual panels stack vertically. On wide screens and in landscape mode, the dual panels arrange horizontally. Typography fluidly adapts to balance font size and available screen size.
History of AMS MathViewer
AMS MathViewer evolved from several prototypes developed at the American Mathematical Society over the past few years, all of which explored various types of presentation for AMS content on the web. In particular, AMS MathViewer's visual design is heavily inspired by AMS Lens, which was developed in collaboration with Substance.io, the developers behind the original eLife Lens project. AMS MathViewer was developed from scratch in collaboration with krautzource.
AMS MathViewer does not attempt to mimic print. Instead, it is based on the web's individual character as a medium: rich document structure, universal access, adaptable display, dynamic interaction, and ease of use. It simplifies "casual uses" such as quickly browsing a new publication or looking up a specific item for reference, while the PDF remains the version of record. In short, AMS MathViewer is not about replacing print and print-like products, rather it focuses on the web's strength as the most universal medium available today.

